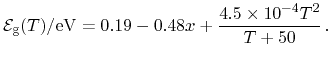5.3.1 Band Gap

Figure 5.8: Temperature dependence of and transition between direct and indirect band gaps in lead telluride.

At room temperature, the band-gap is defined by the direct distance between the valleys at the L-point of the Brillouin zone. In contrast to many other semiconductors, the temperature dependence of this band gap is positive, meaning that with increasing temperatures the direct band gap at the L-point also increases. Beside the valence band maximum at the L point, there exists another one close to $\Sigma$ resulting in an additional indirect band gap separated by $0.14\,\ensuremath{\mathrm{eV}}$ at $0\,\ensuremath{\mathrm{K}}$ [258]. Reported measurement values differ only slightly, where the $0\,\ensuremath{\mathrm{K}}$ band gap is given as $0.19\,\ensuremath{\mathrm{eV}}$ [194]. The gradient describing the temperature dependence in a linear fit is reported to be $4\times10^{-4}\,\ensuremath{\mathrm{K^{-1}}}$ [253,234], $4.1\times10^{-4}\,\ensuremath{\mathrm{K^{-1}}}$ [259], and $4.2\times10^{-4}\,\ensuremath{\mathrm{K^{-1}}}$ [194]. There is a transition between the direct and the indirect band gap at about $420\,\ensuremath{\mathrm{K}}$ depending on the chosen temperature dependence of the direct band gap. The indirect band gap has a value of $0.36\,\ensuremath{\mathrm{eV}}$ which is reported to be temperature independent [191,253].

Several models found in literature have been compared to collected measurement data [255,260,261,199], as illustrated in Fig. 5.8. While the ansatz proposed by Grisar [260] delivers

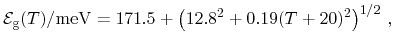$$\ensuremath{\ensuremath{\mathcal{E}}_{\ensuremath{\mathrm{g}}}}(\... ...rm{meV}} = 171.5 + \left( 12.8^2 + 0.19 (\ensuremath{T}+20)^2 \right)^{1/2} \,,$$ (5.21)

Sitter [253] proposed a simple linear fit

$$\ensuremath{\ensuremath{\mathcal{E}}_{\ensuremath{\mathrm{g}}}}(\ensuremath{T})/\ensuremath{\mathrm{eV}} = 0.19 + 4\times10^{-4} \,T \,.$$ (5.22)

The parameters for Varshni's model [262], which is widely used in semiconductor device simulation, have been identified in [202]. Thus, the expression for the band gap for the Varshni model reads

$$\ensuremath{\ensuremath{\mathcal{E}}_{\ensuremath{\mathrm{g}}}}(\... ...{eV}} = 0.19 + \frac{4.5\times10^{-4} \ensuremath{T}^2}{\ensuremath{T}+ 50} \,.$$ (5.23)

Figure 5.9: Temperature dependence of and transition between direct and indirect band gaps in lead tin telluride at tin contents of 0.07 and 0.15.

In PbSnTe, the crossover between the highest valence band and the lowest conduction band throughout a variation of the alloy composition between PbTe and SnTe strongly affects the band gap. This results in decreasing band gap values with increasing SnTe content for PbTe-rich samples, followed by a zero band-gap zone, and finally approaching the SnTe value for lower temperatures. The SnTe content for which the zero band-gap situation occurs shifts to higher contents with increasing temperature [256]. The temperature dependence of the band gap in tin telluride was subject of intense discussion. While generally a slight negative temperature coefficient is suggested, the temperature coefficient's sign is reported to change for higher carrier concentrations [188]. However, for technologically relevant PbTe-rich samples, this is not the case. The band gap models valid for this range are formulated by an extension of the according PbTe models by a material composition dependent expression. Thus, Varshni's extended model reads

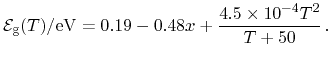$$\ensuremath{\ensuremath{\mathcal{E}}_{\ensuremath{\mathrm{g}}}}(\... ....19 - 0.48 x + \frac{4.5\times10^{-4} \ensuremath{T}^2}{\ensuremath{T}+ 50} \,.$$ (5.24)

An extension to Grisar's model is obtained analogously as

$$\ensuremath{\ensuremath{\mathcal{E}}_{\ensuremath{\mathrm{g}}}}(\... ... = 171.5 - 535 x + \left( 12.8^2 + 0.19 (\ensuremath{T}+20)^2 \right)^{1/2} \,.$$ (5.25)

Fig. 5.9 illustrates the situation for different material compositions. The direct band gap is shifted to lower values with increasing SnTe content until the band inversion occurs. Additionally, the indirect band gap decreases due to a shift of the second valence band. The values identified for $x=0.07$ and $x=0.15$ are $0.29\,\ensuremath{\mathrm{eV}}$ and $0.27\,\ensuremath{\mathrm{eV}}$ , respectively. Measurement data published in [263,254,255,199,264,188,265] have been used to identify the parameters.

M. Wagner: Simulation of Thermoelectric Devices