Integral Equations

4.9 Integral Equations

The integral equation under consideration in this context is the Fredholm integral equation of the second kind, which is commonly given in the form

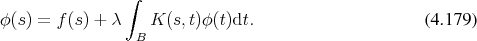
∫ ϕ(s) = f(s) + λ K (s,t)ϕ (t)dt. (4.179) B

$K(s,t)$ is the kernel of the integral equation. As the solution $ϕ(s)$ appears on both sides of Equation 4.179, it can be inserted into itself, thus yielding

∫ b ( ∫ ) ϕ(s)=f(s) + λ K (s,t) f(s) + λ K (s,t)ϕ (t)dt dt = (4.180a) a B ∫ ∫ ( ∫ ) =f(s) + λ K (s,t)f(s)dt + λ2 K (s,t) K (s,t)ϕ(t)dt dt. (4.180b) B B B

Thus the function $ϕ (s)$ takes the form of a series

∞ 2 ∑ i ϕ(s) = ϕ0(s) + ϕ1(s)λ + ϕ2(s)λ + ...= ϕi(s)λ (4.181) i=0

where

∫ ϕn = K (s,t)ϕn −1(t)dt ϕ0(s) = f(s). (4.182) B

While this recursion relation is straightforward, an explicit expression for the $ϕn (s)$ depending only on $ϕ0(s)=f(s)$ is favourable. It can be obtained by rewriting the recursion with a focus on the kernels instead of the functions to read.

∫ ϕn = Kn (s,t)f(t)dt (4.183) B

Now the $Kn(s,t)$ are given as

∫ Kn (s,t) = Kn −1(s,t1)K (t1,t)dt K1 (s,t) = K (s,t) (4.184) B

which can be generalized to

∫ Kp+q (s,t) = Kp (s,t1)Kq(t1,t)dt. (4.185) B

This representation is known as iterated kernels. The resulting series is known as a Neumann series or the resolvent series of the Equation 4.179 and takes the form

2 ∞∑ i R(s,t,λ) = K1 (s,t) + K2 (s,t)λ + K3(s,t)λ + ...= Ki+1 (s,t)λ . (4.186) i=0

Thus the expression of Equation 4.181 may also be expressed as

∫ ∞ ∫ ∑ i ϕ(s)=f(s) + λ B R(s,t,λ)f (t)dt = f (s ) + λ B Ki+1(s,t)λ f(t)dt. (4.187) i=0