Tomas Hadamek MSc Publications

Biography Tomáš was born in Opava, Czech Republic, in 1992. He received his Bachelor degree in Mechatronics from Brno University of Technology in 2015. After an exchange year at the Norwegian University of Science and Technology, Norway, he enrolled in a Master program therein, receiving his Master's degree (MSc) in Physics in November 2018. In addition to his expertise in condensed-matter physics, Tomáš has previous experience working in the fields of numerical acoustics and robotics. Tomáš joined the Institute for Microelectronics am in July 2020, where he is working towards his doctoral degree in micromagnetic simulations for non-volatile magnetic memory devices.

Current memory technology has reached its limits due to rapidly increasing leakage currents caused by transistor scaling and conductor spacing. Moreover, the current von Neumann architecture, which separates memory and computational units, has an intrinsic communication bottleneck and high energy consumption due to the high data transfer rates required for operation. One of the more promising memory candidates to mitigate both problems, spin transfer torque magnetoresistive random access memory (STT-MRAM), is intrinsically non-volatile, highly durable, has broad temperature operation range, and can be integrated into logics due to its complementary metal-oxide semiconductor (CMOS) technology compatibility.

Even though STT-MRAM has already been used in real applications, proper computer-aided simulation tools are yet to be developed. Due to the high current densities passing through STT-MRAM during switching, considerable heat is produced inside the memory cell, and its temperature can significantly increase. The elevated temperatures can mediate switching, but can also dramatically reduce the data retention of the cell. Therefore, proper temperature modeling must be included in simulations. Due to non-uniform magnetization in the memory cell, inhomogeneity of the current density is induced, resulting in non-uniform heating and inhomogeneous temperature in the cell. To simulate these effects, we utilize the so-called Landau–Lifshitz–Gilbert equation (LLGE) coupled to spin, charge, and heat dynamic.

We consider a STT-MRAM cell consisting of a magnetic tunnel junction (MTJ) — an oxide layer sandwiched between two magnetic layers, broadening contacts, and a passivation layer around the MTJ and the narrow parts of the contacts. Fig. 1 shows a temperature profile of such a cell at 2 ns after the beginning of switching from anti-parallel to parallel state of the magnetization layers. The contact ends (bottom and top) are kept at a constant temperature of 300 K. The warmest spot is near the MTJ — the middle of the cell — where the majority of the heat is produced. The left part of the figure shows the temperature super-imposed on the structure itself, with partial opacity to visualize the structure. Fig. 2 shows the temperature development in the free magnetization layer of the MTJ. The temperature differences (maximum, minimum, and average) with respect to the ambient temperature are shown. During switching, significant temperature inhomogeneity develops in the free layer. Fig. 3 shows effects of the non-zero temperature (300 K) on switching. Due to the randomness of the thermal contribution to the LLGE, significant switching differences exist for the different realizations of the same experiment (50 shown in the picture with dashed lines). A switching without temperature field is also shown (solid blue).

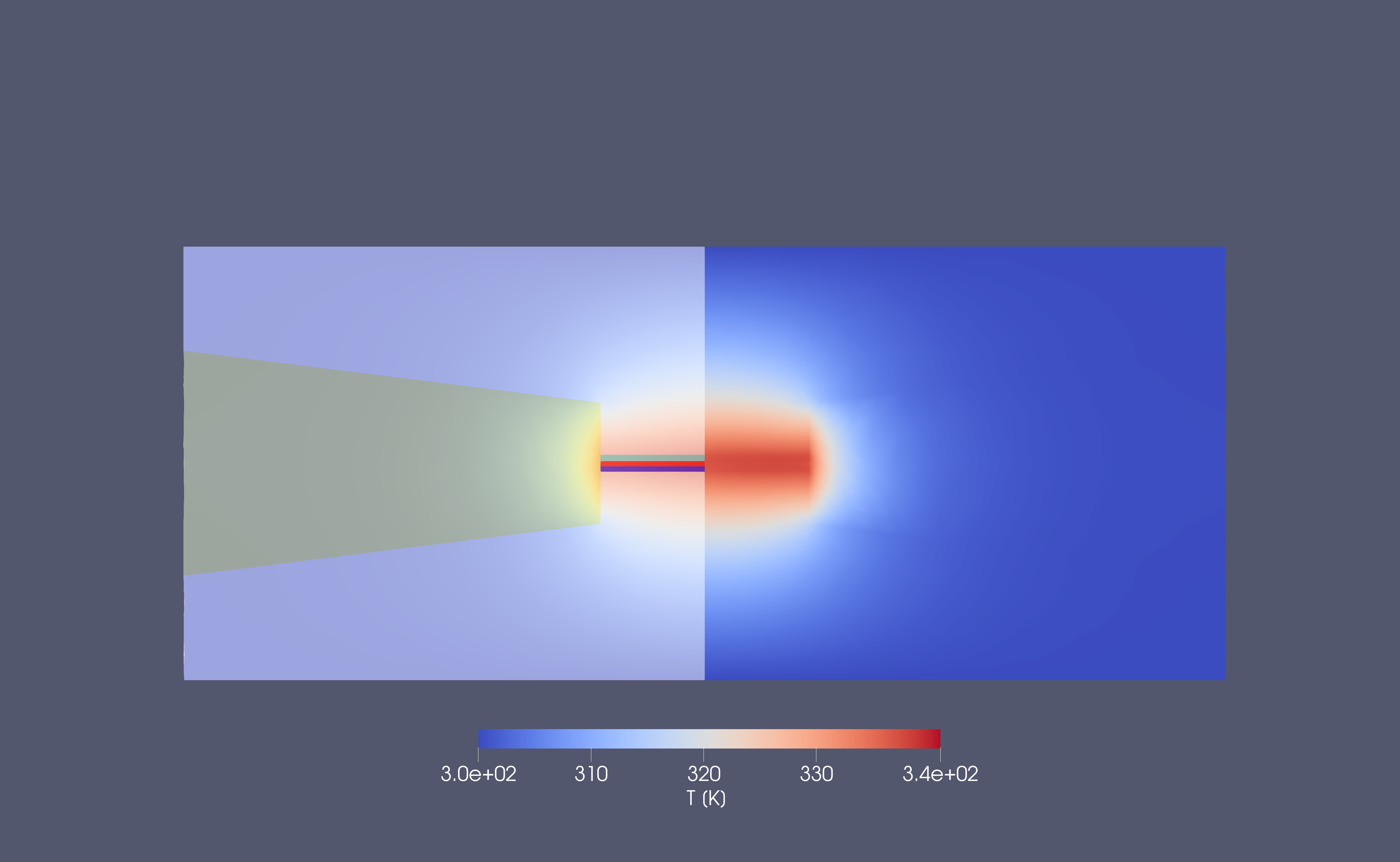
Fig. 1: Temperature of STT-MRAM cell during switching at 2 ns. The cell consists of an MTJ (turquoise, red, blue), broadened contacts (gray) and a passivation layer (yellow). The ends (bottom and top) are kept at a constant temperature of 300 K. A voltage pulse of 2V is applied between the contacts. The majority of the heat is generated near the MTJ (middle of the picture), the temperature increase is also around the MTJ region.

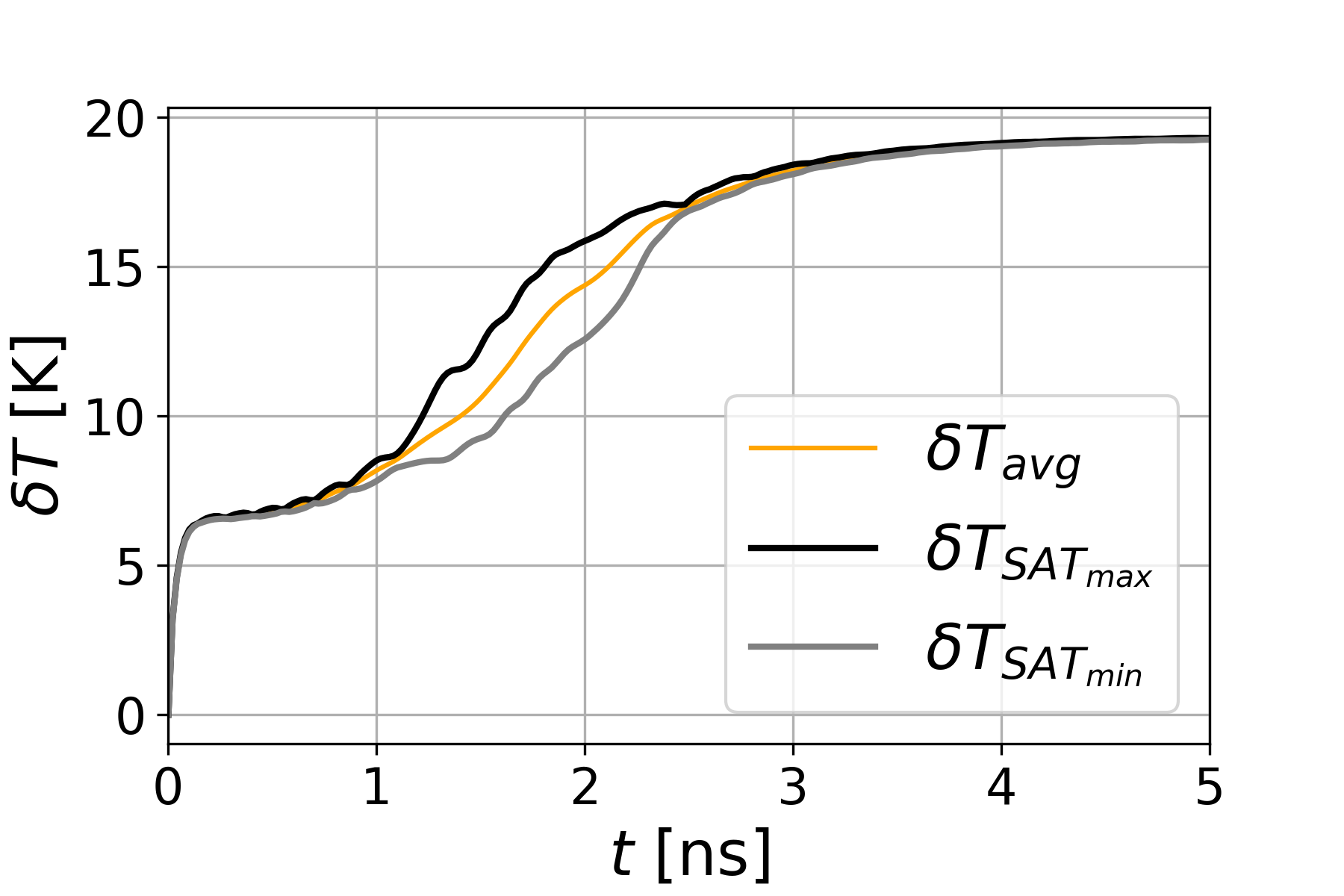
Fig. 2: Temperature increase in the free magnetic layer of the MTJ for 1 V applied over the structure. Temperature differences at the free layer — maximal (black), minimum (gray), average (orange) — with respect to the ambient temperature (300 K) are shown.

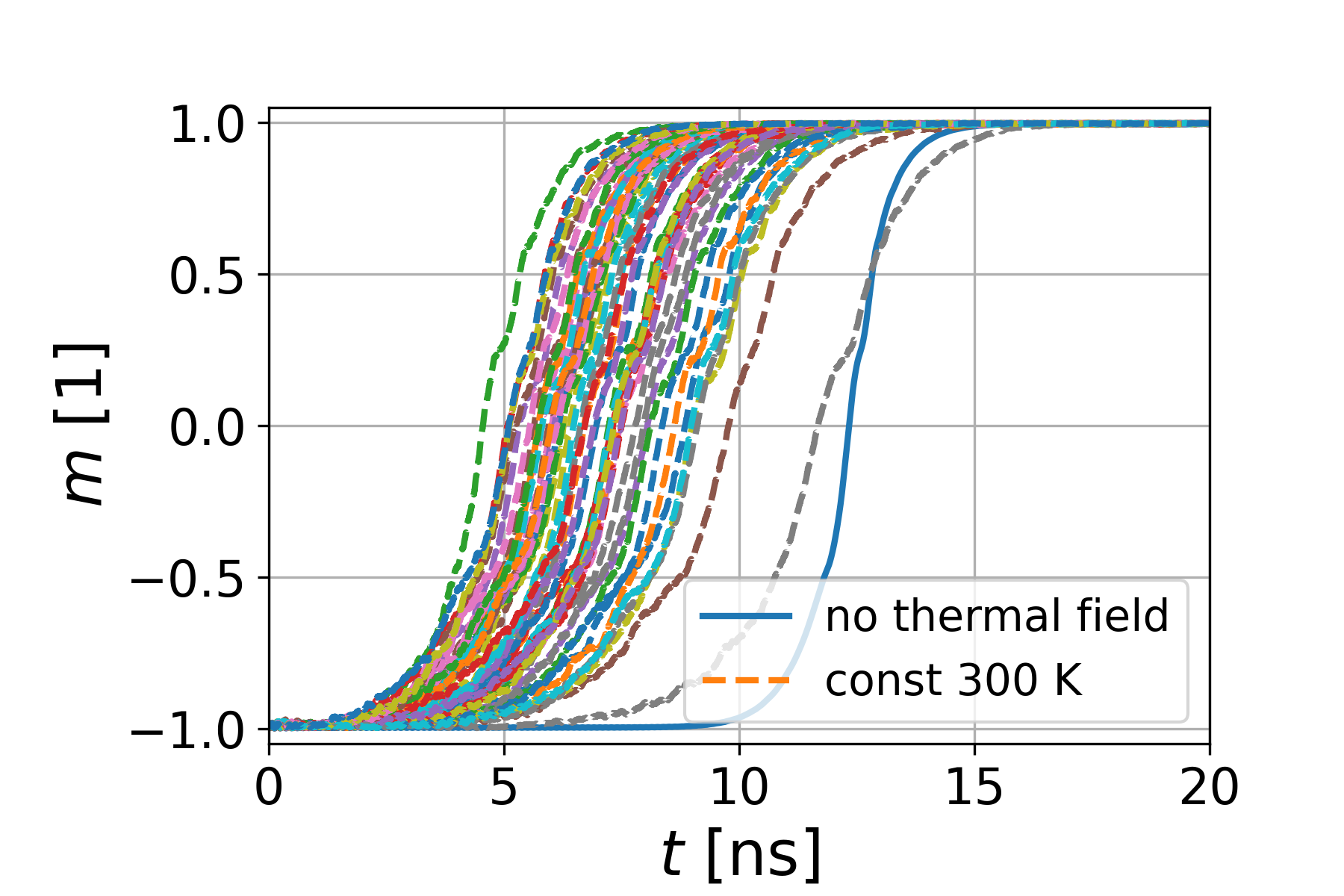
Fig. 3: Switching simulations with thermal field included (displayed with dashed lines). 50 realizations are shown. A simulation without the thermal field is also shown (solid blue).