Johann Cervenka Senior Scientist Dipl.-Ing. Dr.techn. Publications

Biography Johann Cervenka was born in Schwarzach, Austria, in 1968. He studied electrical engineering at the Technische Universität Wien, where he received the degree of Diplomingenieur in 1999. He then joined the Institute for Microelectronics at the Technische Universität Wien and received his PhD in 2004. His scientific interests include three-dimensional mesh generation, as well as algorithms and data structures in computational geometry.

Developing novel nanoelectronic devices requires methods capable of simulating quantum mechanical effects in the carrier transport processes. The Wigner formalism is convenient for the simulation of electronic devices by describing quantum mechanics in the phase space.

Challenges arise in discretizing the diffusion term in the differential equation because of rapid variations of the Wigner function in the phase space. The developed approach uses an integral formulation of the Wigner equation, which mitigates these difficulties.

During the ongoing development of the deterministic method, a new formulation of the evolution in phase space is under examination. The previously used method describes the evolution of fundamental wave packages in phase space during time, summing them up to the entire solution. This method shows high demands when calculating the solutions for each step. With the newly developed method, the evolution of the entire distribution by a Lawson predictor-corrector scheme offers a promising approach.

The method involves two main stages: prediction and correction. In the prediction stage, the scheme utilizes an explicit method to estimate the next state of the system based on the current state. This prediction provides an initial approximation of the solution. Subsequently, in the correction stage, the scheme adjusts the predicted solution iteratively. The corrected solution is then used as the final approximation for the next time step. The entire distribution in phase space can be extended in a forward scheme without the requirement of solving the large equation systems. It can provide more accurate solutions while maintaining computational efficiency.

Future investigations must be carried out to improve the method's performance and examine its stability. The figure shows the first results of the Wigner function in phase space of a propagating wave package reaching a potential barrier. In contrast to the previous simulations, requiring several computation nodes in parallel, the new simulations can run on a single compute node.

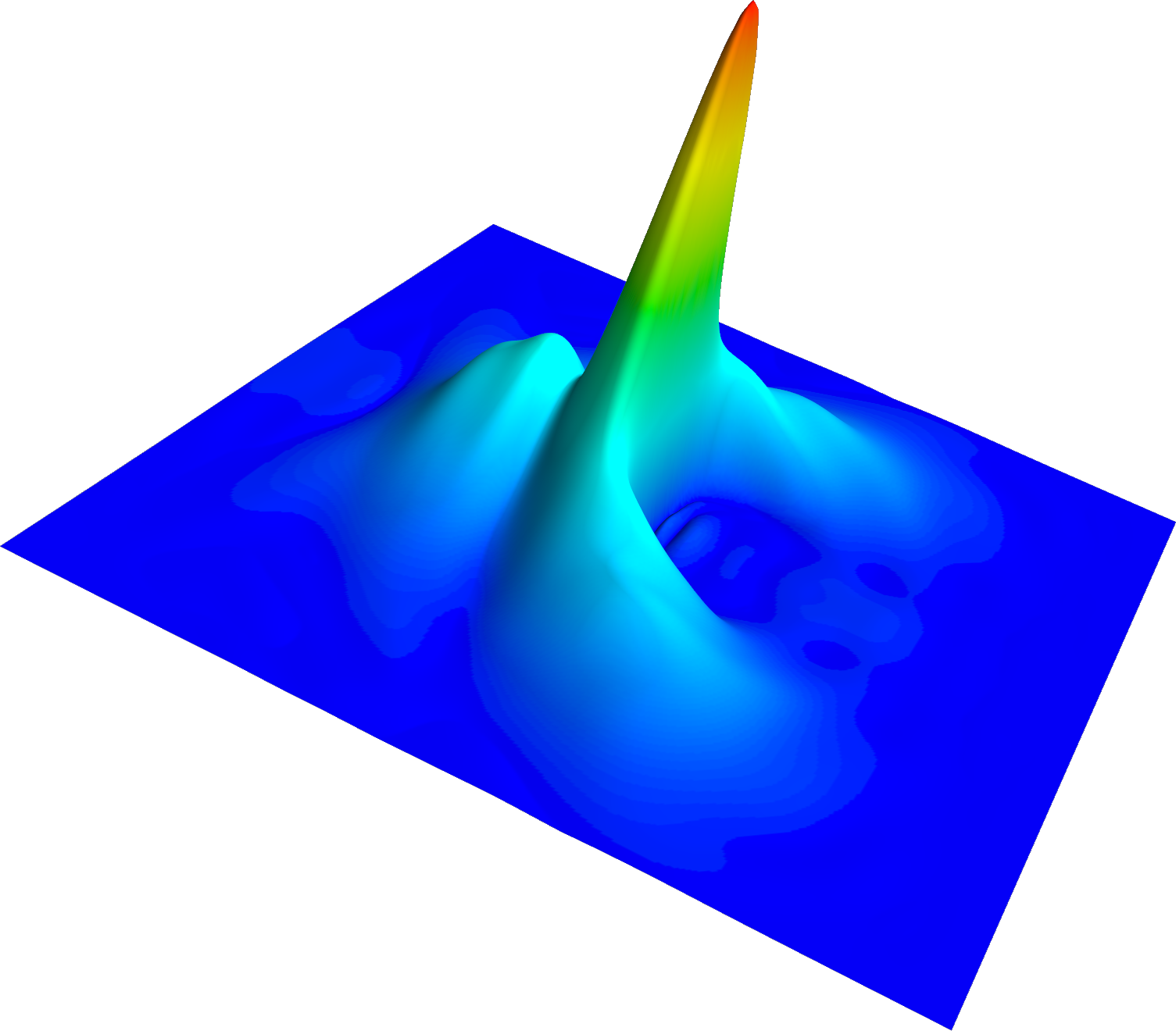
Fig. 1: Density plot of the Wigner function of a wave package reaching a potential barrier in phase space.