



Next: 4.2 Interface Models Up:
4 Description of the Simulator Previous:
4 Description of the Simulator
4.1 Volume Models
In all semiconductor segments Poisson's equation and the continuity
equation for each simulated carrier type have to be solved. Poisson's equation,
derived from the third Maxwell equation, reads:
 , , |
(16)
|
where  is the total electric charge density,
is the total electric charge density,  denotes the displacement vector, q is the electronic charge, n
and p are the electron and hole concentrations,
denotes the displacement vector, q is the electronic charge, n
and p are the electron and hole concentrations,  and
and  are
the concentrations of ionized donors and acceptors, and
are
the concentrations of ionized donors and acceptors, and  is the concentration of ionized deep traps.
is the concentration of ionized deep traps.
The continuity equation for electrons and holes can be derived taking
the zero order momentum of the Boltzmann equation [44,
45]:
 |
(17)
|
 . . |
(18)
|
Taking the first order momentum, the Boltzmann equation yields the
current densities for electron and holes:
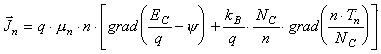 |
(19)
|
 . . |
(20)
|
Here, NC and NV are the effective densities
of states in the conduction and valence band respectively, EC
and EV denote the conduction and the valence band edges,
and µn and µp are the mobilities
of electrons and holes, respectively. These parameters account for different
materials. kB is the Boltzmann constant. If the temperature
Tn of electrons and Tp of holes are
constant, i. e. equal to the lattice temperature, (19)
and (20) reduce to the equations for drift diffusion.
For a hydrodynamic model Tn and Tp
have to be taken into account. In this case the energy transport equations
obtained from the second order momentum of the Boltzmann equation
 |
(21)
|
and
 |
(22)
|
have to be added, where
 |
(23)
|
and
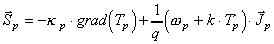 |
(24)
|
are the energy fluxes for electrons and holes,  and
and  are
the energy relaxation times, and
are
the energy relaxation times, and  and
and  are
the energy of electrons and holes, respectively [6].
At room temperature the drift kinetic energy of the carriers is relatively
low compared to the random kinetic energy of the carriers and can be neglected.
Therefore the approximation
are
the energy of electrons and holes, respectively [6].
At room temperature the drift kinetic energy of the carriers is relatively
low compared to the random kinetic energy of the carriers and can be neglected.
Therefore the approximation
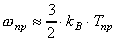 |
(25)
|
for the carrier energy can be used. According to the WiedemannFranz
law the thermal conductivities of electrons and holes are calculated by
 . . |
(26)
|




Next: 4.2 Interface Models Up:
4 Description of the Simulator Previous:
4 Description of the Simulator
Helmut Brech
1998-03-11
 ,
, ,
,
 .
.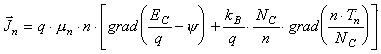
 .
.


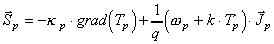
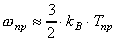
 .
.![]()
![]()
![]()
![]()