Subsections

• 2.2.1 Theory
• 2.2.2 Results and Discussion

2.2 Carrier Concentration Dependence of Mobility

Recently it has been realized that the carrier concentration plays an important role for the mobility. Experiments show that for a hole-only diode and a FET fabricated from the same conjugated polymer, the mobility could differ up to three orders of the magnitude [41]. This difference can only be explained by taking into account the dependence of mobility on the carrier concentration. Rubel [42] analyzed this problem with the concept of a transport energy $E_t$, but there is no direct proof for the existence of such transport energy in organic systems. In this work we will focus on extending the percolation model based on VRH theory by Vissenberg [43] to explain the discrepancy of mobilities measured in OLEDs and OFETs.

In this section, an analytical mobility model with a Gaussian DOS function has been obtained. It can explain the relation between the mobility and carrier concentration. Results are in good agreement with experimental data.

2.2.1 Theory

To calculate the mobility of an organic semiconductor, one can use percolation theory, regarding such system as a random resistor network (network of Miller and Abrahams) [7,44]. The current flows through the bonds connecting the sites in the network. The conductance between the states $m$ and $m'$ can be described as

$$Z_{mm'}^{-1}= Z_0^{-1}\exp\left(-2\alpha\mid R_m-R_{m'}\mid\right... ...-\frac{\mid E_m-E_F\mid+\mid E_{m'}-E_F\mid+\mid E_{m'}-E_m\mid}{2k_BT}\right),$$

where $Z_0^{-1}$ is a prefactor, $\alpha^{-1}$ is the Bohr radius of the localized wave functions, $T$ is the temperature, $R_m$ and $E_m$ denote the position and energy of site $m$. In theory the value of $Z_{mm'}$ is determined by the threshold or critical conductance $Z_c$, at which the first infinite cluster will form, given by the relation

$$\sigma=\sigma_0Z_c^{-1}.$$ (2.1)

Here $\sigma_0$ is a prefactor. To describe the field-effect mobility in organic transistors, Vissenberg assumed an exponential density of localized states [43].

$$g\left(E\right)=\frac{N_t}{k_BT_0}\exp\left(\frac{E}{k_BT_0}\right) \quad\quad\left(E\leq 0\right)$$ (2.2)

$N_t$ is the number of states per unit volume and $T_0$ specifies the width of the exponential distribution. Connecting (2.1) and (2.2), the conductivity can be described as [43]

$$\sigma\left(\delta,T\right)=\sigma_0\left(\frac{\pi\delta N_t\lef... ...ight)^3B_c\Gamma\left(1-T_0/T\right)\Gamma\left(1+T_0/T\right)}\right)^{T_0/T}.$$ (2.3)

Here $B_c$ is the critical number of bonds per site and $\delta$ is the fraction of occupied states, defined as

$$\delta\cong\exp\left(\frac{\epsilon_F}{k_BT_0}\right)\Gamma\left(1-T/T_0\right)\Gamma\left(1+T/T_0\right),$$

$\Gamma$ is the gamma function. Then an expression for the mobility as a function of the carrier concentration $n$ can be obtained.

$$\mu\left(n,T\right)=\frac{\sigma_0}{q}\left(\frac{\left(T_0/T\rig... ...in\left(\pi T/T_0\right)}{\left(2\alpha\right)^3B_c}\right)^{T_0/T}n^{T_0/T-1}.$$ (2.4)

However, this expression can not account for the carrier concentration independent mobility when the carrier concentration is very low (LED regime). To overcome this problem, we derive another mobility model assuming a Gaussian DOS [9] and VRH theory. In this model, the DOS function is given as

$$g\left(E\right)=\frac{N_t}{\sqrt{\pi}k_BT_{\sigma}}\exp\left[-\left(\frac{E}{k_BT_{\sigma}}\right)^2\right].$$ (2.5)

Here $E$ is the energy measured relative to the center of the DOS and $T_{\sigma}$ indicates the width of the DOS. The value of the Fermi energy $E_F$ can be determined by the equation for the carrier concentration $n$.

$$n=\int_{-\infty}^{\infty}\frac{g(E)dE}{1+\exp\left(\left(E-E_F\right)/{k_BT}\right)}.$$ (2.6)

At low concentration, the exponential function is large compared to one (the nondegenerate case) [45], and we obtain the Fermi energy as

$$E_F=-\frac{k_BT_{\sigma}^2}{4T}+k_BT\ln\delta .$$ (2.7)

According to percolation theory [17], at the onset of percolation, the critical number $B_c$ can be written as

$$B_c=\frac{N_b}{N_s}.$$ (2.8)

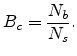$B_c=2.8$ for a three-dimensional amorphous system, $N_b$ and $N_s$ are, respectively, the density of bonds and density of sites in a percolation system, which can be calculated as [43,46]

$$N_b=\int d{\bf {R}}_{ij}dE_idE_jg\left(E_i\right)g\left(E_j\right)\theta\left(s_c-s_{ij}\right)$$

and

$$N_s=\int dE g\left(E\right)\theta\left(s_ck_BT-\mid E-E_F\mid\right).$$

Here ${\bf R_{ij}}$ denotes the distance vector between sites $i$ and $j$, $s_c$ is the exponent of the conductance given by the relation $\sigma=\sigma_0e^{-s_c}$ [13] and $\theta$ is step function.
Substituting (2.5) and (2.7) into (2.8), we obtain a new percolation criterion for an organic system as

$$B_c\approx\frac{2N_t\left(\sqrt{2}+1\right)\sqrt{\pi}}{\left(2\al... ...p\left(-\left[\frac{E_F+k_BTs_c}{k_BT_{\sigma}}\right]^2\right).\quad\quad\quad$$

This equation has to be solved for $s_c$ and an expression for mobility can be obtained.

$${\mu=\frac{\sigma_0}{qN_t}\exp\left(\eta\right)},$$ (2.9)

where

$$\eta=-\frac{T_\sigma}{T}\sqrt{-W\left[-\frac{B_c\left(2\alpha T/T... ...gma\right)^3}{2\pi N_t\left(1+\sqrt{2}\right)}\right]} -\frac{T_\sigma^2}{4T^2}$$

$W$ is the Lambert function [47]. Equation (2.9) is obtained assuming

• that the site positions are random,
• the energy barrier for the critical hop is large,
• and the charge carrier concentration is very low.

Figure 2.1: Comparison between the analytical model (2.9) and empirical model $\mu \approx \textrm { exp } \left (-\left (C\sigma /{k_BT}\right )^2\right )$ for different temperature.

Figure 2.2: The mobility as a function of $\left (T_\sigma /T\right )^{1/3}$ for different $\alpha$.

a

b

Figure 2.3: Fermi-energy as a function of the carrier occupation probability. The symbols represent Fermi-Dirac and the solid lines Boltzmann represent statistics. Panel (a) shows the case of carrier occupation between $10^{-40}$ and 1. Panel (b) shows the case of carrier occupation bigger than $10^{-10}$.

(a)

(b)

Figure 2.4: The calculated mobility versus carrier occupation at different temperature.

Figure 2.5: Comparison between calculation and typical experimental results [41].

2.2.2 Results and Discussion

So far, much attention has been devoted to explain the temperature dependence of the mobility [48,49,50]. As shown in Fig 2.1, the model (2.9) gives a non-Arrhenius-type temperature dependence of the form $\mu\propto\exp\left(-\left(C\sigma/k_BT\right)^2\right)$, which has also been supported by numerical simulations [51] and analytical calculations [53]. The model (2.9) shows good agreement for a value $C\approx 0.71$. This value is close to $C\approx 0.69$ given in [52] and $0.64$ in [53].

In Fig 2.2, the mobility is plotted as a function of $(T_\sigma/T)^{1/3}$. When plotted in this way, there exists the regime with a linear relation between $\mu$ and $T^{-1/3}$. This indicates that the variable-range hopping effect has to be taken into account [54,55].

To obtain (2.7), a Boltzmann distribution function has been used. The degenerate limit of organic semiconductors has been studied in [56,57]. In Fig 2.3 (a) we show the Fermi energy for Boltzmann and Fermi-Dirac distributions assuming some typical values of the parameter 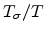$T_\sigma/T$ as 1.5, 3.5 and 6.0 [48]. Fig 2.3 (b) is a comparison especially for the higher carrier occupation regime. The analytical result (2.7) agrees well with the numerically calculated result for decreasing carrier occupation and increasing 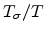$T_\sigma/T$. Therefore, for the LED regime with low charge carrier concentration, (2.7) is a good approximation of the solution of (2.6).

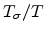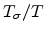
The mobility as a function of the carrier concentration is presented in Fig 2.4, where $T_\sigma/T$ is in the range $1.5-9.0$, corresponding to some typical values for organic semiconductors. The mobility stays constant until a certain threshold value of the carrier occupation. Above this threshold, the mobility can increase about four orders of magnitude at $T_\sigma/T$$=$ 9. These effects have also been observed experimentally [41,58].

However, (2.9) is valid only in the LED regime with very low carrier concentration. As it is difficult to get an analytical expression for the mobility at higher carrier concentration, we use (2.4) as the mobility model for the higher carrier concentration. The combined model can explain the experimental data in [41,58], as shown in Fig 2.5.