3.4.1 Lattice Mobility

At finite temperature the lattice atoms oscillate about their equilibrium sites. The carriers are scattered and this results in a temperature dependent mobility $\mu^{\mathrm{L}}$. The superscript L stands for lattice scattering. The temperature dependence of the lattice mobility is modeled by a power law for all semiconductors.

$$\mu^{\mathrm{L}}_{\nu} = \mu^{\mathrm{L}}_{\nu,300}\cdot\lef... ...mathrm{300 K}}\right)^{\gamma_{0,\nu}}, \hspace{1cm}\nu = n,p$$ (3.96)

The parameter values used in this model for electrons and holes, respectively, are summarized in Table 3.21.

Table 3.21: Parameter values for the lattice mobility

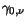Material	$\nu$	$\mu^{\mathrm{L}}_{\nu,300}$ [cm$^2$/Vs]	$\gamma_{0,\nu}$	Range	References
Si	n	1430	-2	1500	[86,90,85]
	p	460	-2.18	450-500	[86,90,85]
Ge	n	3800	-1.66	3900	[86,90,85,161]
	p	1800	-2.33	1900	[86,90,85,161]
GaAs	n	8500	-2.2	8500-9400	[86,117,85,162]
	p	800	-0.9	400-492	[86,117,85,162]
AlAs	n	410	-2.1	400-1200	[162,117,85]
	p	130	-2.2	130-420	[93,117,85]
InAs	n	32500	-1.7	22600-34000	[117,86,92,162]
	p	510	-2.3	200 - 530	[117,86,92,162]
InP	n	5300	-1.9	4200-5400	[117,86,91]
	p	200	-1.2	130-180	[117,86,85]
GaP	n	210	-1.94	110-300	[86,92,91,85]
	p	160	-2.0	75-150	[86,117]