2.2 Theoretical Background

The structure of CNTs has been explored early by high resolution transmission electron microscopy techniques yielding direct confirmation that the CNTs are seamless cylinders derived from the honeycomb lattice representing a single atomic layer of crystalline graphite, called a graphene sheet. The structure of a SW-CNT is conveniently explained in terms of its one-dimensional unit cell, defined by the vectors ${\bf C_h}$ and ${\bf T}$ as shown in Fig. 2.2.

The circumference of any CNT is expressed in terms of the chiral vector ${\bf C_h}=n{\bf a_1}+m{\bf a_2}$ which connects two crystallographically equivalent sites on a two-dimensional graphene sheet [16]. The construction in Fig. 2.2 depends uniquely on the pair of integers $(n,m)$ which specify the chiral vector. The chiral angle $\theta$ is defined as the angle between the chiral vector ${\bf C_h}$ and the zigzag direction ($\theta =0$). Three distinct types of CNT structures can be generated by rolling up the graphene sheet into a cylinder as shown in Fig. 2.3. The zigzag and armchair CNTs correspond to chiral angles of $\theta =0$ and $\theta =30$°, respectively, and chiral CNTs correspond to $0<\theta <30$°. The intersection of the vector $\overrightarrow{OB}$ (which is normal to ${\bf C_h}$) with the first lattice point determines the fundamental one-dimensional translation vector ${\bf T}$. The unit cell of the one-dimensional lattice is the rectangle defined by the vectors ${\bf C_h}$ and ${\bf T}$.

The cylinder connecting the two hemispherical caps of the CNT (see Fig. 2.3) is formed by superimposing the two ends of the vector ${\bf C_h}$ and the cylinder joint is made along the two lines $\overrightarrow{OB}$ and $\overrightarrow{AB'}$ in Fig. 2.2. The lines $\overrightarrow{OB}$ and $\overrightarrow{AB'}$ are both perpendicular to the vector ${\bf C_h}$ at each end of ${\bf C_h}$ [16]. In the $(n,m)$ notation for ${\bf C_h}=n{\bf a_1}+m{\bf a_2}$, the vectors $(n,0)$ or $(0,m)$ denote zigzag CNTs, whereas the vectors $(n,m)$ correspond to chiral CNTs [21]. The CNT diameter $d_\mathrm{CNT}$ is given by

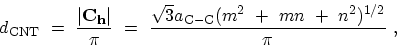$$\begin{array}{l}\displaystyle d_\mathrm{CNT} \ = \ \frac{\ver... ..._\mathrm{C-C}(m^2\ +\ mn \ + \ n^2)^{1/2}}{\pi} \ , \end{array}$$ (2.1)

where $\vert{\bf C_h}\vert$ is the length of ${\bf C_h}$ and $a_\mathrm{C-C}$ is the C-C bond length (1.42 Å). The chiral angle $\theta$ is given by $\theta \ = \ \mathrm{tan}^{-1}[\sqrt{3}n/(2m\ + \ n)]$. For the $(n,n)$ armchair CNT $\theta =30$° and for the $(n,0)$ zigzag CNT $\theta=60$°. From Fig. 2.2 it follows that if one limits $\theta$ to the range $0\leq\theta\leq30$°, then by symmetry, $\theta =0$ for a zigzag CNT. Both armchair and zigzag CNTs have a mirror plane and thus are considered achiral. Differences in the CNT diameter $d_\mathrm{CNT}$ and chiral angle $\theta$ give rise to different properties of the various CNTs. The number $N$ of hexagons per unit cell of a CNT, specified by integers $(n,m)$, is given by

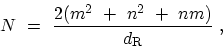$$\begin{array}{l}\displaystyle N \ = \ \frac{2(m^2 \ + \ n^2 \ +\ nm)}{d_\mathrm{R}} \ , \end{array}$$ (2.2)

where $d_\mathrm{R}=d$ if $n-m$ is not a multiple of $3d$, and $d_\mathrm{R}=3d$ if $n-m$ is a multiple of $3d$, and $d$ is defined as the greatest common divisor (gcd) of $(n,m)$. Each hexagon in the honeycomb lattice contains two carbon atoms. The unit cell area of the CNT is $N$ times larger than that for a graphene layer and consequently the unit cell area for the CNT in reciprocal space is correspondingly $1/N$ times smaller. Table 2.2 provides a summary of relations useful for describing the structure of SW-CNTs [12,22].

Figure 2.2: The chiral vector ${\bf C_h}=n{\bf a_1}+m{\bf a_2}$ is defined on the honeycomb lattice of carbon atoms by unit vectors ${\bf a_1}$ and ${\bf a_2}$ and the chiral angle $\theta$ with respect to the zigzag axis ($\theta =0$). The diagram is constructed for $(n,m)=(4,2)$.

Figure 2.3: Schematic models of SW-CNTs with the CNT axis normal to the chiral vector. The latter is along (a) the $\theta =30$° direction for an $(n,n)$ armchair CNT, (b) the $\theta =0$ direction for a $(n,0)$ zigzag CNT, and (c) a general $\theta$ direction with $0<\theta <30$° for a $(n,m)$ chiral CNT.

Table 2.2: Structural properties for CNTs [12].

Symbol	Description	Formula
a	length of unit vectors	$a=\sqrt{3}a_\mathrm{C-C}=2.49$ Å,     $a_\mathrm{C-C}=1.42$ Å
${\bf a_1}$, ${\bf a_2}$	unit vectors	$$\left(\frac{\sqrt{3}}{2},\frac{1}{2}\right)a,~\left(\frac{\sqrt{3}}{2}, -\frac{1}{2}\right)a$$
${\bf b_1}$, ${\bf b_2}$	reciprocal lattice vectors	$$\left(\frac{1}{\sqrt{3}},1\right)\frac{2\pi}{a},~ \left(\frac{1}{\sqrt{3}},-1\right)\frac{2\pi}{a}$$
${\bf C_h}$	chiral vector	${\bf C_h}=n{\bf a_1}+m{\bf a_2}\equiv(n,m)$,     $(0\leq\vert m\vert\leq n)$
$L$	length of ${\bf C_h}$	$L=\vert{\bf C_h}\vert=a\sqrt{n^2+m^2+nm}$
$d_\mathrm{CNT}$	diameter	$$d_\mathrm{CNT}=\frac{L}{\pi}$$
$\theta$	chiral angle	$$\mathrm{tan}(\theta)=\frac{\sqrt{3}}{2n+m}$$
$d$	gcd$(n,m)$
$d_\mathrm{R}$	gcd $(2n+m,2m+n)$
${\bf T}$	translational vector	$$\begin{array}{l}\displaystyle \hspace*{-30pt}{\bf T}=t_1{\bf ... ...+n}{d_\mathrm{R}},~~t_2=-\frac{2n+m} {d_\mathrm{R}} \end{array}$$
$T$	length of ${\bf T}$	$T=\vert{\bf T}\vert=\displaystyle \frac{\sqrt{3}L}{d_\mathrm{R}}$
$N$	number of hexagons in the unit-cell	$$N = \frac{2(n^2+m^2+mn)}{d_\mathrm{R}}$$

M. Pourfath: Numerical Study of Quantum Transport in Carbon Nanotube-Based Transistors