4.2.4 The Resolvent Series

Equation (4.24) represents a Fredholm integral equation of second kind with a free term determined by function $G(\vec{k})$. This equation can be rewritten^4.4:

$$f(x)=\int f(x^{'})K(x^{'},x)\,dx^{'}+g(x),$$ (4.25)

where the $K(x^{'},x)$ and the free term are given functions. The multidimensional variable $x$ stands for $(\vec{k},t)$ ^4.5. The resolvent series^4.6 of a Fredholm integral equation is obtained by replacement of its right hand side into itself:

$$f(x)=g(x)+\int g(x_{1})K(x_{1},x)\,dx_{1}+\int dx_{1}\int dx_{2}\,g(x_{1})K(x_{1},x_{2})K(x_{2},x)+\cdots$$ (4.26)

This means that the solution of (4.24) can be written as consecutive iterations of the free term:

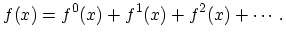$$f(x)=f^{0}(x)+f^{1}(x)+f^{2}(x)+\cdots.$$ (4.27)

To find the iteration terms explicitly (4.24) is rewritten as:

$$f_{1}(\vec{k},t)= \int_{0}^{t}\,dt_{1}\int \,d\vec{k}_{1}f_{1}(\vec{k}_{1},t_{1})\w... ...))\cdot \exp\biggl(-\int_{t_{1}}^{t}\widetilde{\lambda}[\vec{K}(y)]\,dy\biggr)+$$

$$+G(\vec{K}(0))\exp\biggl(-\int_{0}^{t}\widetilde{\lambda}[\vec{K}(y)]\,dy\biggr),$$ (4.28)

where $(\vec{k}^{'},t^{'})$ has been replaced by $(\vec{k}_{1},t_{1})$ and the respective quasi-momentum space trajectory is:

$$\vec{K}(t_{1})=\vec{k}-\frac{q}{\hbar}\vec{E}_{s}(t-t_{1}),$$

$$\vec{K}(t)=\vec{k},$$ (4.29)

$$0<t_{1}<t.$$

Introducing a quasi-momentum space trajectory for the time interval $0<t_{2}<t_{1}$:

$$\vec{K}_{1}(t_{2})=\vec{k}_{1}-\frac{q}{\hbar}\vec{E}_{s}(t_{1}-t_{2}),$$

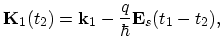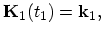$$\vec{K}_{1}(t_{1})=\vec{k}_{1},$$ (4.30)

one obtains for $f_{1}(\vec{k}_{1},t_{1})$:

$$f_{1}(\vec{k}_{1},t_{1})= \int_{0}^{t_{1}}\,dt_{2}\int \,d\vec{k}_{2}f_{1}(\vec{k}_{2},t_{2... ...\exp\biggl(-\int_{t_{2}}^{t_{1}}\widetilde{\lambda}[\vec{K}_{1}(y)]\,dy\biggr)+$$

$$+G(\vec{K}_{1}(0))\exp\biggl(-\int_{0}^{t_{1}}\widetilde{\lambda}[\vec{K}_{1}(y)]\,dy\biggr).$$ (4.31)

Substituting (4.32) into (4.29) gives:

$$f_{1}(\vec{k},t)=\int_{0}^{t}\,dt_{1}\int\,d\vec{k}_{1}\int_{0}^{... ...biggl(-\int_{t_{2}}^{t_{1}}\widetilde{\lambda}[\vec{K}_{1}(y)]\,dy\biggr)\times$$

$$\times\widetilde{S}(\vec{k}_{1},\vec{K}(t_{1}))\exp\biggl(-\int_{t_{1}}^{t}\widetilde{\lambda}[\vec{K}(y)]\,dy\biggr)+$$ (4.32)

$$+\int_{0}^{t}\,dt_{1}\int\,d\vec{k}_{1}G(\vec{K}_{1}(0))\exp\bigg... ...(t_{1}))\exp\biggl(-\int_{t_{1}}^{t}\widetilde{\lambda}[\vec{K}(y)]\,dy\biggr)+$$

$$+G(\vec{K}(0))\exp\biggl(-\int_{0}^{t}\widetilde{\lambda}[\vec{K}(y)]\,dy\biggr).$$

From (4.34) the first iteration term is obtained:

$$f_{1}^{(1)}(\vec{k},t)=$$

$$\int_{0}^{t}\,dt_{1}\int\,d\vec{k}_{1}G(\vec{K}_{1}(0))\exp\biggl... ...(t_{1}))\exp\biggl(-\int_{t_{1}}^{t}\widetilde{\lambda}[\vec{K}(y)]\,dy\biggr).$$ (4.33)

which is also schematically shown in Fig. 4.1.

Figure 4.1: Graphical representation of the first iteration term.

In order to obtain the second iteration term the third quasi-momentum space trajectory is introduced for $0<t_{3}<t_{2}$

$$\vec{K}_{2}(t_{3})=\vec{k}_{2}-\frac{q}{\hbar}\vec{E}_{s}(t_{2}-t_{3}),$$

$$\vec{K}_{2}(t_{2})=\vec{k}_{2}.$$ (4.34)

Then for $f_{1}(\vec{k}_{2},t_{2})$ in (4.32) one obtained:

$$f_{1}(\vec{k}_{2},t_{2}) =\int_{0}^{t_{2}}\,dt_{3}\int \,d\vec{k}_{3}f_{1}(\vec{k}_{3},t_{... ...\exp\biggl(-\int_{t_{3}}^{t_{2}}\widetilde{\lambda}[\vec{K}_{2}(y)]\,dy\biggr)+$$

$$+G(\vec{K}_{2}(0))\exp\biggl(-\int_{0}^{t_{2}}\widetilde{\lambda}[\vec{K}_{2}(y)]\,dy\biggr).$$ (4.35)

The second iteration term is obtained from (4.36) by replacing $f_{1}(\vec{k}_{3},t_{3})$ with the free term of (4.29):

$$f_{1}^{(2)}(\vec{k},t)=\int_{0}^{t}\,dt_{1}\int\,d\vec{k}_{1}\int... ...exp\biggl(-\int_{0}^{t_{2}}\widetilde{\lambda}[\vec{K}_{2}(y)]\,dy\biggr)\times$$

$$\times\widetilde{S}(\vec{k}_{2},\vec{K}_{1}(t_{2}))\exp\biggl(-\i... ...(t_{1}))\exp\biggl(-\int_{t_{1}}^{t}\widetilde{\lambda}[\vec{K}(y)]\,dy\biggr).$$ (4.36)

This term is displayed graphically in Fig. 4.2.

Figure 4.2: Graphical representation of the second iteration term.

S. Smirnov: