4.1.3 Substrate Orientation (110)

On (110) oriented substrate, the principal crystallographic system does not coincide with a system where the $z$ axis is normal to the substrate surface and a coordinate transformation is necessary to obtain the quantization and transport masses. From the direction of the substrate normal

$${\ensuremath{\mathitbf{e}}}_n^{(110)} = \frac{1}{\sqrt 2}(1,1,0) = (\cos\phi\sin\theta, \sin\phi\sin\theta, \cos\theta)\ ,$$ (4.19)

the angles $\theta=90^\circ$ and $\phi=45^\circ$ of the coordinate transformation can be identified. The transformation matrix becomes

$$\ensuremath{{\underaccent{\bar}{U}}}= \begin{pmatrix}0 & -1/\sqrt 2 & 1/\sqrt 2 \\ 0 & 1/\sqrt 2 & 1/\sqrt 2 \\ -1 & 0 & 0 \end{pmatrix}.$$ (4.20)

The inverse effective mass tensors of the three valley pairs can be calculated from (4.6)

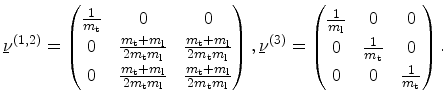$$\ensuremath{{\underaccent{\bar}{\nu}}}^{(1,2)}= \begin{pmatrix}\f... ...m_\mathrm{t}}} & 0\\ 0 & 0 & \frac{1}{\ensuremath{m_\mathrm{t}}} \end{pmatrix}.$$ (4.21)

Figure 4.3: (a) Alignment of constant-energy surfaces of the Si conduction band with respect to the substrate surface (110). (b) Projection of constant-energy surfaces onto the (110) plane. Concentric ellipses (unfilled) indicate the fourfold degeneracy of the unprimed ladder. The constant-energy lines belonging to the primed ladder are filled in dark grey.

         [a] [b]

It can be seen that the quantization masses $\smash{m^{(1,2)}_{\perp} = 1 / \nu^{(1,2)}_{33} = 2 \ensuremath{m_\mathrm{l}}\ensuremath{m_\mathrm{t}}/(\ensuremath{m_\mathrm{t}}+\ensuremath{m_\mathrm{l}})}$ for the two valley pairs labeled $1$ and $2$ in Figure 4.3a are equal. The quantization mass of the remaining two valleys (labeled $3$ in Figure 4.3a) is $m^{(3)}_{\perp}=\ensuremath{m_\mathrm{t}}$. Since $2 \ensuremath{m_\mathrm{l}}\ensuremath{m_\mathrm{t}}/(\ensuremath{m_\mathrm{t}}+\ensuremath{m_\mathrm{l}}) > \ensuremath{m_\mathrm{t}}$, the four valleys with the larger quantization mass $m^{(1,2)}_{\perp}$ belong to the lowest (unprimed) subband ladder, whereas the two valleys with $m^{(3)}_\perp=\ensuremath{m_\mathrm{t}}$ constitute the primed subband ladder. To calculate the transport masses the eigenvalue problem given in (4.17) for $\ensuremath{{\underaccent{\bar}{M}}}^v$ has to be solved. On (110) oriented substrate $\ensuremath{{\underaccent{\bar}{M}}}^v$ is given by

$$\ensuremath{{\underaccent{\bar}{M}}}^{(1,2)} = \begin{pmatrix}\fr... ...ath{m_\mathrm{l}}} & 0\\ 0 & \frac{1}{\ensuremath{m_\mathrm{t}}} \end{pmatrix}.$$ (4.22)

Thus, the transport masses of the unprimed subband ladder

$$m^{(1,2)}_{\shortparallel,1} = \ensuremath{m_\mathrm{t}}\ ,\quad ... ...{\shortparallel,2} = (\ensuremath{m_\mathrm{l}}+\ensuremath{m_\mathrm{t}})/2\ ,$$ (4.23)

and the primed ladder

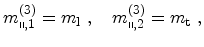$$m^{(3)}_{\shortparallel,1} = \ensuremath{m_\mathrm{l}}\ ,\quad m^{(3)}_{\shortparallel,2} = \ensuremath{m_\mathrm{t}}\ ,$$ (4.24)

can be obtained. In Figure 4.3b the constant-energy lines of the subbands for a (110) oriented substrate are shown. The unprimed ladder is fourfold degenerate, whereas the primed ladder is twofold degenerate. This is opposite to the situation for (001) oriented substrate, where the unprimed ladder was twofold degenerate and the primed ladder was fourfold degenerate. The major principal axis of the unprimed subbands is $[\bar{1}10]$, whereas the major principal axis of the twofold degenerate primed subband ladder is [001].

E. Ungersboeck: Advanced Modelling Aspects of Modern Strained CMOS Technology