5.3.1 Bulk Scattering Mechanisms

The collision integral at the right hand side of Boltzmann's transport equation is defined as the rate of change of the distribution function

$$\biggl(\frac{\partial f_n}{\partial t}\biggr)_\mathrm{coll} = \frac{\Omega}{(2\pi)^3} \sum_{n'} \int_{\mathrm{BZ}} (1-f_n({\e... ...hitbf{r}}},t)f_{n'}({\ensuremath{\mathitbf{r}}},{\ensuremath{\mathitbf{k}}}',t)$$

$$-(1-f_{n'}({\ensuremath{\mathitbf{r}}},{\ensuremath{\mathitbf{k}}... ...{r}}},t) f_n({\ensuremath{\mathitbf{r}}},{\ensuremath{\mathitbf{k}}},t)d^3k'\ .$$ (5.7)

It describes the transition from an arbitrary state $(n', {\ensuremath{\mathitbf{k}}}')$ into the state $(n, {\ensuremath{\mathitbf{k}}})$ and the reverse process assuming that scattering does not change the particle's spin. The probability density rate for a transition from the initial state $(n', {\ensuremath{\mathitbf{k'}}})$ to a final state $(n, {\ensuremath{\mathitbf{k}}})$ depends on position and is proportional to the occupancy of the initial state $f_{n'}({\ensuremath{\mathitbf{r}}},{\ensuremath{\mathitbf{k'}}})$, proportional to the transition rate $S_{nn'}({\ensuremath{\mathitbf{k}}},{\ensuremath{\mathitbf{k}}}',{\ensuremath{\mathitbf{r}}},t)$, and to the probability that the final state is not occupied $(1-f_n({\ensuremath{\mathitbf{r}}},{\ensuremath{\mathitbf{k}}}))$. The latter factor is a result of the Pauli Principle and will be discussed in more detail in Section 5.4.

Omitting time and position dependence for the sake of brevity, the scattering rates are defined as

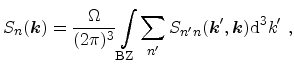$$S_{n}({\ensuremath{\mathitbf{k}}}) = \frac{\Omega}{(2\pi)^3} \int... ...n}({\ensuremath{\mathitbf{k'}}},{\ensuremath{\mathitbf{k}}}) \mathrm{d}^3 k'\ ,$$ (5.8)

where the integration can be performed over the first BZ or any primitive cell in the reciprocal space. The scattering rate represents the rate at which particles are scattered out of the initial state $(n, {\ensuremath{\mathitbf{k}}})$. From Fermi's Golden Rule it follows that the scattering rates are proportional to the final density of states per spin, $g_{n}\left(E\right)$, which is given by

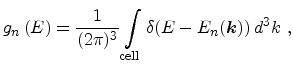$$g_{n}\left(E\right)=\frac{1}{(2\pi)^{3}}\int_{\mathrm{cell}}\delta(E-E_{n}({\ensuremath{\mathitbf{k}}}))\,d^{3}k\ ,$$ (5.9)

In fullband MC simulations this integral is evaluated numerically. Using an analytical description for the conduction bands, the minima of the conduction bands (valleys) are approximated using the bandform function

$$\gamma^v(E) = \frac{\hbar^2}{2} \sum_{i,j=1}^3 k_i \frac{1}{m^{*v}_{ij}} k_j = \left (E^v_{\mathrm{nonpar}}({\ensuremath{\mathitbf{k}}}) - E^v... ...+\alpha^{v} (E^v_{\mathrm{nonpar}}({\ensuremath{\mathitbf{k}}}) - E^v_0)\right)$$ (5.10)

$$= E \left( 1 + \alpha^{v} E\right)$$ (5.11)

Note that the band index $n$ was replaced by the valley index $v$, and $E$ denotes the energy with respect to the valley offset $E_0$. $m^{*v}_{ij}$ denotes the effective mass tensor, and $\alpha^{v}$ the nonparabolicity coefficient of the valley with index $v$. A parabolic band dispersion is obtained if the nonparabolicity coefficient $\alpha^v$ is zero.

The density of states of the analytical band structure evaluates to

$$g^{v}\left(E\right)=\frac{1}{\sqrt{2}} \frac{\{m_\mathrm{dos}^v\}^{3/2}}{\pi^{2}\hbar^{3}}\sqrt{\gamma^{v}\left(E\right)}(1+2\alpha^{v}E)\ ,$$ (5.12)

where $m^v_{\mathrm{dos}}$ denotes the density of states mass of the $v$-th valley

$$m^v_\mathrm{dos}= \sqrt[3]{ \{m^*_{11}\}^v \{m^*_{22}\}^v \{m^*_{33}\}^v } \ ,%\vphantom{\sum_i}$$ (5.13)

which can be calculated from the effective mass tensor.

The transition rates from state ( $v,{\ensuremath{\mathitbf{k}}}$) to state ( $v',{\ensuremath{\mathitbf{k}}}'$) for phonon scattering in a non-polar semiconductor can be written as [Jacoboni83]

$$\{\tiny S^{ \shortstack{abs \\ [-2pt] emi }} \} ^{v'v}({\ensurema... ...ath{\mathitbf{k}}}') - E^{v}({\ensuremath{\mathitbf{k}}}) \mp \hbar\omega_q]\ .$$ (5.14)

Here, the upper and lower symbols refer to phonon absorption and emission, respectively. The rate depends on the momentum transfer ${\ensuremath{\mathitbf{q}}} = {\ensuremath{\mathitbf{k}}} - {\ensuremath{\mathitbf{k}}}'$, the phonon number $N_q$, the deformation potential tensor $\mathcal{D}_{ij}$ , the mass density of the crystall $\rho$, the phonon angular frequency $\omega_q$ , its polarization $\xi_i$, and the overlap integral $\mathscr{O}$,

$$\mathscr{O} = \left \vert\,\int_{BZ} u_{{\ensuremath{\mathitbf{k}... ...ensuremath{\mathitbf{G}}}\cdot{\ensuremath{\mathitbf{r}}})d^3r\right \vert^2\ .$$ (5.15)

The overlap factors depend on the type of transition. For intravalley transitions of electrons, $\mathscr{O}$ is frequently set to unity, even though this is true only for exact plane waves or for wave functions formed with pure $s$ states [Jacoboni83]. Because the lowest conduction band of cubic semiconductors is a mixture of a $s$ and $p$-type state, an overlap factor less than unity is obtained. Both for intra- and intervalley transitions $\mathscr{O}$ was found to be almost constant for each type of scattering process [Reggiani73], thus the values for $\mathscr{O}$ may be included in the coupling constants.

Subsections

• Acoustic Intravalley Scattering
• Optical Intravalley Scattering
• Intervalley Scattering

E. Ungersboeck: Advanced Modelling Aspects of Modern Strained CMOS Technology