Next: 3.4 Miller Index Notation Up: 3. Strain and Semiconductor Previous: 3.2 Stress

3.3 Stress-Strain Relation

Within the elastic limit of a material Hooke's law is a good approximation for relating stress and strain The generalization of Hook's law for three-dimensional elastic bodies leads to

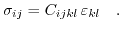$\displaystyle \sigma_{ij}=C_{ijkl}\,\varepsilon_{kl}\quad.$

(3.10)

The elastic stiffness tensor $C_{ijkl}$ is of fourth order and contains

( $3^{4}$ ) elements. Introducing additional symmetry considerations, the number of needed components can be reduced [162]. Cubic semiconductors like

or $G\!aAs$ are characterized by only three constants ( $c_{11}, c_{12}, \mathrm{and}\: c_{44}$ ).

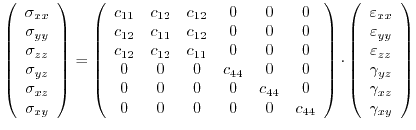$\displaystyle \left(\begin{array}{c} \sigma_{xx}\\ \sigma_{yy}\\ \sigma_{zz} \\... ...\ \varepsilon_{zz}\\ \gamma_{yz}\\ \gamma_{xz}\\ \gamma_{xy} \end{array}\right)$

(3.11)

If the strain, instead of stress, is the quantity of interest, it can be calculated by inversion of the elastic stiffness tensor (3.10)

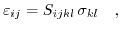$\displaystyle \varepsilon_{ij}=S_{ijkl}\, \sigma_{kl}\quad,$

(3.12)

or in matrix form

$\displaystyle \left(\begin{array}{c} \varepsilon_{xx}\\ \varepsilon_{yy}\\ \var... ...y}\\ \sigma_{zz} \\ \sigma_{yz}\\ \sigma_{xz} \\ \sigma_{xy} \end{array}\right)$

(3.13)

The stiffness constants are normally denoted as $c_{ij}$ , while the compliance constants are named as $s_{ij}$ . The compliance constants can be calculated from the stiffness constants with the following relations:

$\begin{displaymath}\begin{array}{cc} s_{11}=&\frac{c_{11}+c_{12}}{c_{11}^{2}+c_{... ...\quad,\mathrm{and}\\ s_{44}=&\frac{1}{c_{44}}\quad. \end{array}\end{displaymath}$

(3.14)

Next: 3.4 Miller Index Notation Up: 3. Strain and Semiconductor Previous: 3.2 Stress

T. Windbacher: Engineering Gate Stacks for Field-Effect Transistors