 |
|
||||
BiographyHans Kosina received the Diplomingenieur degree in electrical engineering and PhD from the Technische Universität Wien in 1987 and 1992, respectively. He was with the Institute of Flexible Automation at the Technische Universität Wien for one year and then joined the Institute for Microelectronics, where he is currently an associate professor. He received the venia docendi in microelectronics in 1998. In the summer of 1993, he was a visiting scientist at Motorola Inc., Austin, Texas, and in the summer of 1999, a visiting scientist at Intel Corp., Santa Clara, California. Dr. Kosina was chairman of the ''11th International Workshop on Computational Electronics'' held in Vienna in May 2006 and is a member of the advisory board of the International Workshop on Computational Nanotechnology. He has served as an Associate Editor of the IEEE Transactions on Computer-Aided Design of Circuits and Systems and the Journal of Computational Electronics. His current research interests include nanoelectronic device modeling and the development of numerical methods and algorithms for classical and quantum transport calculations. |
|||||
Numerical Modeling of Nanostructured Semiconductor Devices
The three main topics investigated this year are transport modeling in the presence of electron-electron scattering, numerical methods for the stationary Wigner and von Neumann equations, and quantum transport models for device TCAD.
The Boltzmann equation becomes non-linear if electron-electron interaction is included in the scattering operator. On the other hand, a two-particle kinetic equation will remain linear in this case. A two-particle Monte Carlo method in a uniform electric field has been developed previously, which is based on the calculation of trajectory pairs and sampling of the six-dimensional momentum-space of two particles. We observe that the mean velocity, mean energy, and the distribution function, as functions of the uniform electric field, are virtually unaffected by electron-electron scattering (EES). This Monte Carlo algorithm has recently been extended to account for spatially varying electric fields. We have studied the energy distribution function of electrons at various locations in a MOSFET channel. Fig. 1 shows a potential profile in a channel with a length of 50 nm, along with a colormap of the distribution function. The jet of electrons injected from the source and its replicas occurring due to phonon emission is clearly visible. Without EES, the distribution function exhibits a thermal tail, whereas EES enhances the tail. This effect is widely assumed in the modeling of hot carrier degradation, but has not been rigorously modeled so far due to the difficulties associated with the non-linearity of the Boltzmann equation.
A project on numerical methods for the stationary Wigner equation and the von Neumann equation is ongoing. The coupled system of the transport equation and the Poisson equation has been studied in further detail. For the sake of computational efficiency, numerical investigations on the physically equivalent Schrödinger-Poisson system have been carried out. This nonlinear system has been solved using bias-stepping, combined with a full Newton method. The k-grid representing the injected Schrödinger modes is refined adaptively. Parallelization is employed for the assembly of the Jacobian. In general, the solution of a nonlinear system need not be unique. For the test case of a resonant tunneling diode, we find four different equilibrium solutions. These solutions lie on four solution segments, connected through bifurcation points. The selected simulation examples numerically demonstrate that the solution of the Schrödinger-Poisson system at a fixed bias is in general not unique, and the global solution structure of the continuation problem is non-trivial.
A project dealing with quantum transport models for device TCAD is ongoing. A ballistic NEGF transport model in the mode space approximation is implemented in the GTS Nano Device Simulator (NDS). For this purpose, a software interface has been developed, which allows the coupling of the quantum transport module to the existing Boltzmann module. This way, also the self-consistent iteration scheme, available in NDS, has been utilized. An implementation of models for mode coupling and dissipative carrier scattering is in progress.
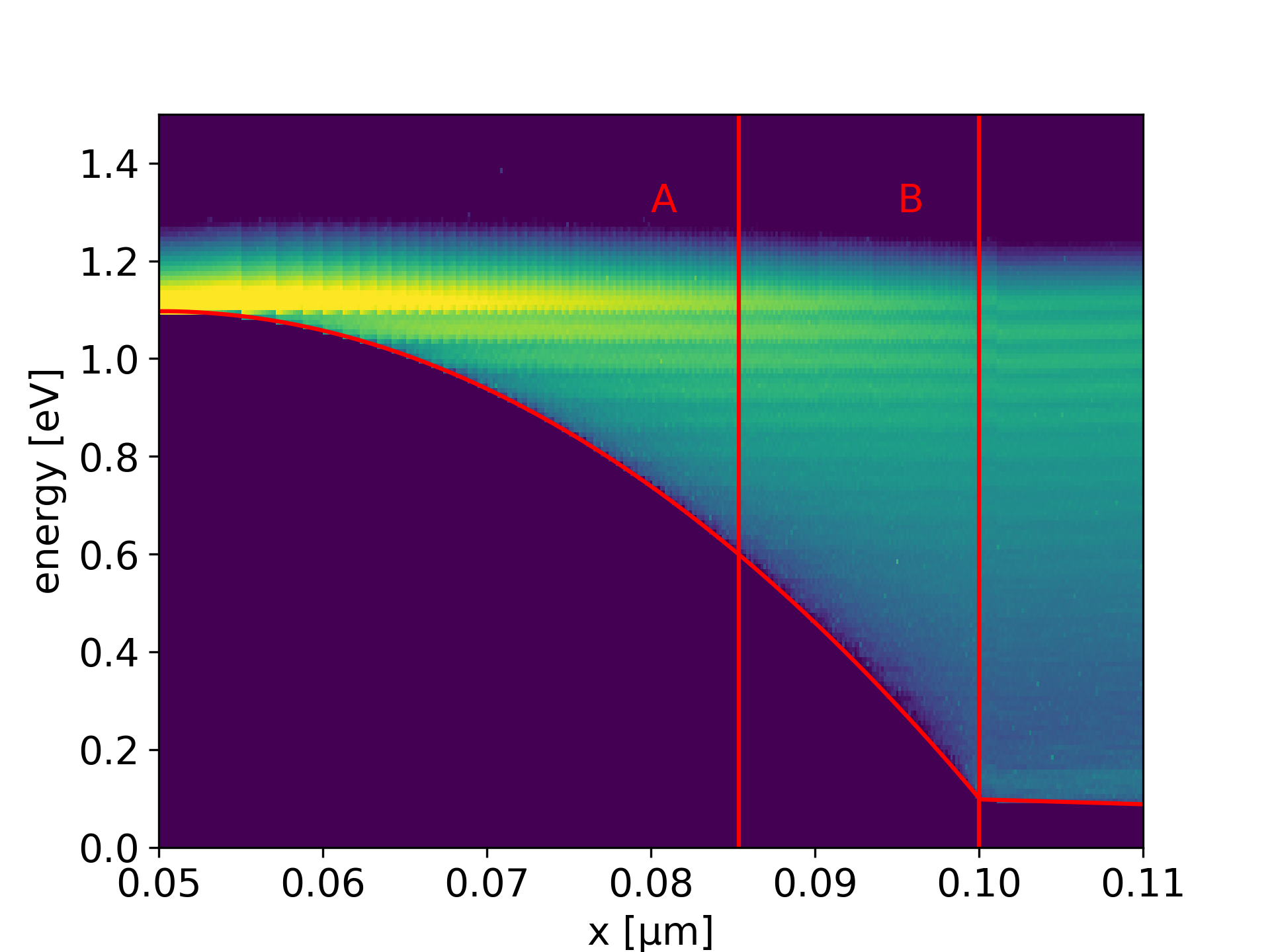
Fig. 1: Potential profile in a 50 nm channel and the electron distribution function. Electron-electron scattering alters the high energy tail of the distribution.


