2.4.2 Classical Device Simulation

If the quantum-mechanical nature of electrons is neglected, carrier transport in a device can be described by BOLTZMANN's transport equation which is a seven-dimensional integro-differential equation in the phase space [46]. For electrons it reads

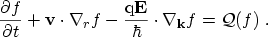$$\frac{\partial f}{\partial t} + {\mathbf{v}} \cdot \ensuremath{{\... ...{E}}}{\hbar} \cdot \ensuremath{{\mathbf{\nabla}}}_{\bf k} f = \mathcal{Q}(f)\ .$$ (2.2)

Here, $f(\mathbf{r},\mathbf{k},t)$ is the distribution of carriers in space ( $\mathbf{r}$), momentum ( $\hbar\mathbf{k}$), and time. On the right-hand side of this partial differential equation stands the collision operator $\mathcal{Q}(f)$ which describes scattering of particles due to phonons, impurities, interfaces, or other scattering sources. However, the direct solution of this equation is computationally prohibitive^2.7. It is rather solved by approximate means applying the method of moments or using methods. In the method of moments each term of (2.2) is multiplied with a weight function and integrated over $\mathbf{k}$-space. This yields a set of differential equations in the ( $\mathbf{r},t$)-space. The moments of the distribution function are defined by [54]

$$\langle \Phi \rangle = \frac{1}{4\pi^3} \int \Phi \, f(\mathbf{r},\mathbf{k},t) \, \ensuremath {\mathrm{d}}^3 k\ .$$ (2.3)

Subsections

• 2.4.2.1 The Drift-Diffusion Model
• 2.4.2.2 The Energy-Transport Model
• 2.4.2.3 Device Simulation

A. Gehring: Simulation of Tunneling in Semiconductor Devices