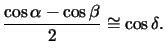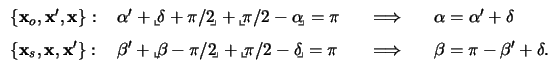A. Approximation of the Inclination Factor

During the derivation of the Fresnel and the Fraunhofer diffraction formulae (4.14) and (4.15), respectively, the inclination factor occurring in (4.10) has been approximated under the assumption that the source x_s as well as the observation point x_o are located near the optical axis (cf. Figure 4.1). We will now explain how this approximation is obtained.

First we define three angles $\alpha$, $\beta$, and $\delta$ guided by Figure 4.1 and shown in Figure A.1:

$$\alpha=\angle(\mathbf{n},\mathbf{x}_o-\mathbf{x}),\qquad\quad\bet... ...}_s-\mathbf{x}),\qquad\quad\delta=\angle(\mathbf{n},\mathbf{x}_o-\mathbf{x}_s).$$ (A.1)

The approximation of the inclination factor--compare (4.12) with (4.14) or (4.15)--thus writes to

 

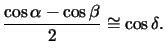$$\frac{\cos\alpha-\cos\beta}{2} \cong \cos\delta.$$ (A.2)

Furthermore we introduce the two auxiliary angles $\alpha^{\prime}_{}$ and $\beta^{\prime}_{}$ as illustrated in Figure A.1. In the situation of interest the two points x_s and x_o are near the optical axis and thus $\alpha^{\prime}_{}$,$\beta^{\prime}_{}$ $\ll$ 1 yielding

 

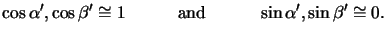$$\cos \alpha^\prime, \cos\beta^\prime \cong 1\quad\qquad\text{and}\qquad\quad\sin \alpha^\prime, \sin\beta^\prime \cong 0.$$ (A.3)

By noting that the sum of the angles of the two triangles {x_o,x^$\prime$,x} and {x_s,x,x^$\prime$} equals $\pi$, we get^a

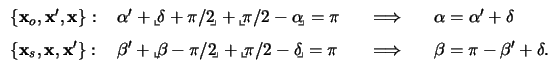$$\begin{array}{llll}\{\mathbf{x}_o,\mathbf{x}^\prime,\mathbf{x}\}:... ... \pi&\quad\Longrightarrow\quad& \beta = \pi - \beta^\prime + \delta.\end{array}$$ (A.4)

With these two expressions for $\alpha$ and $\beta$ and the approximations listed in (A.3), the derivation of (A.2) is straight forward:

$$\cos\alpha - \cos\beta = \cos(\alpha^\prime+\delta) + \cos(\beta^\prime-\delta)$$

$$= \cos\alpha^\prime\cos\delta-\sin\alpha^\prime\sin\delta +\cos\beta^\prime\cos\delta+\sin\beta^\prime\sin\delta$$

$$\cong 2\cos\delta.$$

  

Figure A.1: Approximation of the inclination factor. The dashed lines indicate the two auxiliary triangles used throughout the derivation.

Footnotes

... get^a

The terms forming one common triangle-angle are indicated by delimiters (cf. Figure A.1).