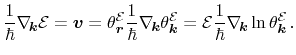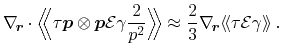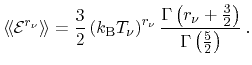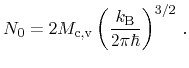3.5.5.2 Particle Flux Equation

In the following, the particle flux equation is derived, whereby the starting point is Boltzmann's equation with a general vector-valued weight $\ensuremath{\ensuremath{\mathitbf{X}}}$ in the form of equation (3.30). For the flux equations, the time derivative (first term in (3.30)) can be safely neglected, since the relaxation time is in the order of picoseconds, which ensures quasi-stationary behavior even for today's fastest signals [87]. This means that a transient signal must only change as fast as the carriers are available to follow into a new equilibrium state.

Inserting $\ensuremath{{\tau}}\ensuremath{\ensuremath{\mathitbf{p}}}$ as $\ensuremath{\ensuremath{\mathitbf{X}}}$ into (3.30) delivers the particle current equation in its original form which serves as a basis for further derivations

$$\underbrace{\vphantom{\frac{1}{\hbar}}\ensuremath{\ensuremath{\en... ...angle \! \langle \ensuremath{\ensuremath{\mathitbf{p}}} \rangle \! \rangle} \,.$$ (3.38)

The single contributions to the left side can be identified as a diffusion term (i) and two drift terms (ii) and (iii) , whereby the latter one is caused by external electric fields (iii) . In the sequel, these terms are subject to several simplifications caused by assumptions on the distribution function, the band structure, and the relaxation time.

Equation (3.38) contains statistical averages of tensor-valued quantities, which are subject to closer investigation in the following. With the assumption of an almost isotropic distribution function, the non-diagonal elements of the tensors are negligible. For a hot, slowly drifting electron gas, such as discussed in Sections 3.4 and 3.5.1, the influence of the displacement on the averages of even weights, such as energy-like tensors is negligible [84]. Thus, each of the terms can be represented by proper scalar quantities, which are expressed using traces of the corresponding tensor-valued transport parameters. For example, $\ensuremath{\ensuremath{\mathitbf{p}}}\ensuremath{\otimes} \ensuremath{\ensuremath{\mathitbf{v}}}$ can be estimated using

$$\ensuremath{\ensuremath{\mathitbf{p}}}\ensuremath{\otimes}\ensure... ...suremath{\mathitbf{v}}}\right)} \ensuremath{\ensuremath{{\mathrm{\hat{I}}}}}\,.$$ (3.39)

Monte-Carlo simulations indicate the validity of this approximation. It turned out that for the case sketched above, the non-diagonal elements are about five magnitudes smaller than the diagonal elements. In low field cases, the assumption of isotropy is fulfilled very well for the materials taken into account in this work.

In order to incorporate the band structure in an analytical way, assumptions on the dispersion relation as discussed in Section 3.3 have to be made. In order to obtain a mathematically convenient formulation, a product ansatz for the kinetic energy separating the dependencies on $\ensuremath {\ensuremath {\mathitbf {r}}}$ and $\ensuremath{\ensuremath{\mathitbf{k}}}$ is performed

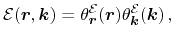$$\ensuremath{\mathcal{E}}(\ensuremath{\ensuremath{\mathitbf{r}}},\... ...itbf{k}}}^\ensuremath{\mathcal{E}}}(\ensuremath{\ensuremath{\mathitbf{k}}}) \,,$$ (3.40)

which will be expressed by parabolic bands later on in this derivation. As a direct consequence, the energy's gradients in $\ensuremath {\ensuremath {\mathitbf {r}}}$ - and $\ensuremath{\ensuremath{\mathitbf{k}}}$ -space read

$$\ensuremath{\ensuremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}}\en... ...math{\theta_\ensuremath{\ensuremath{\mathitbf{r}}}^\ensuremath{\mathcal{E}}}\,,$$ (3.41)

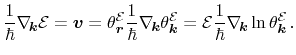$$\ensuremath{\frac{1}{\hbar} \ensuremath{\ensuremath{\mathitbf{\na... ...math{\theta_\ensuremath{\ensuremath{\mathitbf{k}}}^\ensuremath{\mathcal{E}}}\,.$$ (3.42)

It is useful to introduce a non-parabolicity factor $\ensuremath{\gamma}(\ensuremath{\ensuremath{\mathitbf{k}}})$ which becomes $1$ for parabolic bands. Thus, the velocity reads

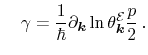$$\ensuremath{\ensuremath{\mathitbf{v}}}= \ensuremath{\mathcal{E}}\... ...thcal{E}}\ensuremath{\gamma}\frac{2\ensuremath{\ensuremath{\mathitbf{p}}}}{p^2} \quad \ensuremath{\gamma}= \ensuremath{\frac{1}{\hbar} \ensuremat... ...suremath{\ensuremath{\mathitbf{k}}}^\ensuremath{\mathcal{E}}}}} \frac{p}{2} \,.$$ (3.43)

In the following, the three parts of equation (3.38) indicated by the horizontal braces are sequentially treated. Applying Eqs. (3.39) and (3.43) to equation (3.38), part (i), one obtains

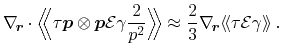$$\ensuremath{\ensuremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}\ens... ...math{{\tau}}\ensuremath{\mathcal{E}}\ensuremath{\gamma} \rangle \! \rangle} \,.$$ (3.44)

For the second part of equation (3.38), the Poisson bracket has to be expanded using (A.10) as well as the definitions for the Poisson bracket, equations (A.1) and (A.6)

$$\ensuremath{\langle \! \langle \ensuremath{\{\ensuremath{{\tau}}\... ...nsuremath{\{\ensuremath{{\tau}},\ensuremath{\mathcal{E}}\}} \rangle \! \rangle}$$ (3.45)

$$= \ensuremath{\Bigl\langle \! \! \Bigl\langle \ensuremath{{\tau}}... ...nabla_{\!r}}}}}\ensuremath{\mathcal{E}}\right) \Bigr\rangle \! \! \Bigr\rangle}$$

$$\quad + \ensuremath{\Bigl\langle \! \! \Bigl\langle \ensuremath{\... ...nabla_{\!r}}}}}\ensuremath{\mathcal{E}}\right) \Bigr\rangle \! \! \Bigr\rangle}$$

The first term vanishes due to the momentum being orthogonal to the space vector, and finally the approximation for tensor valued quantities (3.39) and the application of identity (B.5) leads to

$$\ensuremath{\Bigl\langle \! \! \Bigl\langle -\ensuremath{{\tau}}\... ...nabla_{\!r}}}}}\ensuremath{\mathcal{E}}\right) \Bigr\rangle \! \! \Bigr\rangle}$$ (3.46)

$$= - \ensuremath{\langle \! \langle \ensuremath{{\tau}}\ensuremath... ...emath{\mathitbf{r}}}^\ensuremath{\mathcal{E}}} \Bigr\rangle \! \! \Bigr\rangle}$$

$$= - \ensuremath{\langle \! \langle \ensuremath{{\tau}}\ensuremath... ...math{\theta_\ensuremath{\ensuremath{\mathitbf{r}}}^\ensuremath{\mathcal{E}}}\,.$$

Part (iii) of equation (3.38) has to be converted using identity (B.4) before the trace approximation can be performed, which leads to

$$\mathrm{q}\ensuremath{\Bigl\langle \! \! \Bigl\langle \ensuremath... ...suremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}} \ensuremath{\tilde{\varphi}}\,.$$ (3.47)

Assembling the terms (i) - (iii) again, the isotropic particle current equation with a product ansatz used on the kinetic energy $\ensuremath{\mathcal{E}}$ is obtained

$$\ensuremath{\langle \! \langle \ensuremath{\ensuremath{\mathitbf{... ...uremath{\theta_\ensuremath{\ensuremath{\mathitbf{r}}}^\ensuremath{\mathcal{E}}}$$ (3.48)

$$\quad -\frac{2}{3} \ensuremath{\langle \! \langle \ensuremath{\ma... ...uremath{\theta_\ensuremath{\ensuremath{\mathitbf{r}}}^\ensuremath{\mathcal{E}}}$$

$$\quad + \ensuremath{\mathrm{s}_\nu}\mathrm{q}\ensuremath{\langle ... ...suremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}}\ensuremath{\tilde{\varphi}} \,.$$

In order to obtain a closed formulation, the relaxation time has to be parametrized with macroscopic quantities available in the equation system. Thus, a power-law approximation is introduced as discussed in Section 3.5.4. The according reference energy $\ensuremath{\ensuremath{\mathcal{E}}_0}$ refers to the energy in local thermal equilibrium with the lattice and thus incorporates the lattice temperature. $\ensuremath{{\tau}}$ is expressed as

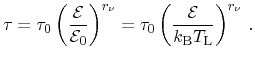$$\ensuremath{{\tau}}= \ensuremath{\tau_0}\left( \frac{\ensuremath{... ...math{\mathrm{B}}\ensuremath{T_{\mathrm{L}}}} \right)^{{\ensuremath{r_\nu}}} \,.$$ (3.49)

Inserting (3.49) to (3.48) yields

$$\ensuremath{\langle \! \langle \ensuremath{\ensuremath{\mathitbf{... ...e \ensuremath{\mathcal{E}}^{{\ensuremath{r_\nu}}+1} \rangle \! \rangle} \right)$$ (3.50)

$$+ \ensuremath{\tau_0}\frac{1}{\ensuremath{\left( k_\ensuremath{\m... ...uremath{\theta_\ensuremath{\ensuremath{\mathitbf{r}}}^\ensuremath{\mathcal{E}}}$$

$$+ \ensuremath{\mathrm{s}_\nu}\mathrm{q}\ensuremath{\tau_0}\frac{1... ...suremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}}\ensuremath{\tilde{\varphi}} \,.$$

In order to close the equation system, a heated, displaced Maxwellian in the diffusion approximation (3.18) is assumed. This approximation is justified by the comparably low drift velocities in thermoelectric devices. Furthermore, parabolic bands (3.13) are introduced

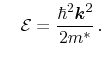$$\ensuremath{f}= \ensuremath{A \left( 1+\frac{\ensuremath{\ensurem... ...th{\mathitbf{k}}})}{k_\ensuremath{\mathrm{B}}\ensuremath{T_\ensuremath{\nu}}}}} \quad \ensuremath{\mathcal{E}}= \frac{\hbar^2 \ensuremath{\ensuremath{\mathitbf{k}}}^2}{2 m^*} \,.$$ (3.51)

With these assumptions, the average in (i) is first transformed to polar coordinates and furthermore to an integral in $\ensuremath{\mathcal{E}}$ -space and the gamma function can be identified. The integral over the odd term of the distribution function

$$\ensuremath{f}_A = A \, \frac{\ensuremath{\ensuremath{\mathitbf{p... ...ath{\mathitbf{k}}})}{k_\ensuremath{\mathrm{B}}\ensuremath{T_\ensuremath{\nu}}}}$$ (3.52)

vanishes, since the product of an even and an odd function results in an odd function

$$\ensuremath{\langle \! \langle \ensuremath{\mathcal{E}}^{{\ensure... ...\mathrm{d}}k_x \, \ensuremath{\,\mathrm{d}}k_y \, \ensuremath{\,\mathrm{d}}k_z}$$ (3.53)

$$=4\pi\ensuremath{A}\ensuremath{\int\limits_{0}^{\infty}\ensuremat... ... \frac{\d\ensuremath{\mathcal{E}}}{\d k} = \frac{\hbar^2 k}{\ensuremath{{m^*}}}$$

$$= \frac{4\pi\sqrt{2}\ensuremath{{m^*}}^{\frac{3}{2}}}{\hbar^3}\en... ...}^{-\ensuremath{\mathcal{E}}}\ensuremath{\,\mathrm{d}}\ensuremath{\mathcal{E}}}$$

$$=\frac{4\pi\sqrt{2}\ensuremath{{m^*}}^{\frac{3}{2}}}{\hbar^3}\ens... ...rac{5}{2}}\ensuremath{\Gamma \left({\ensuremath{r_\nu}}+\frac{5}{2}\right)} \,.$$

The integral for the carrier density is derived analogously, and yields

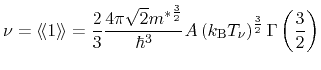$$\ensuremath{\nu}= \ensuremath{\langle \! \langle 1 \rangle \! \ra... ...suremath{\nu}}\right)^{\frac{3}{2}}\ensuremath{\Gamma \left(\frac{3}{2}\right)}$$ (3.54)

whereby the identity $\zeta \ensuremath{\Gamma \left(\zeta\right)} = \ensuremath{\Gamma \left(\zeta + 1\right)}$ has been applied. The carrier concentration is introduced to normalize (3.50). Finally, the carrier mobility is obtained from a coefficient comparison for the homogeneous case as [73]

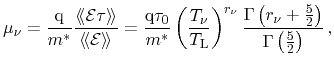$$\ensuremath{\ensuremath{\mu}_\nu}= \frac{\mathrm{q}}{m^*} \frac{\... ...h{r_\nu}}+\frac{5}{2}\right)}}{\ensuremath{\Gamma \left(\frac{5}{2}\right)}}\,,$$ (3.55)

for a heated, displaced Maxwellian. Inserting equations (3.54) and (3.55) to (3.53), part (i) becomes

$$\frac{2}{3} \ensuremath{\ensuremath{\ensuremath{\mathitbf{\nabla_... ...suremath{\mu}_\nu}\ensuremath{\nu}\ensuremath{T_\ensuremath{\nu}}m^*\right) \,.$$ (3.56)

Parts (ii) and (iii) can be treated as described above. The gradient in the second average has to be expanded resulting in the sum of two expressions, a $\ensuremath{\ensuremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}}\ln m^*$ and a $\ensuremath{\ensuremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}}\ensuremath{T_{\mathrm{L}}}$ term. The average needed for the expressions of part (iii) can be derived analogously to (3.53) and results in

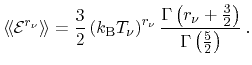$$\ensuremath{\langle \! \langle \ensuremath{\mathcal{E}}^{{\ensure... ...{r_\nu}}+\frac{3}{2}\right)}}{\ensuremath{\Gamma \left(\frac{5}{2}\right)}} \,.$$ (3.57)

Assembling the three parts and inserting the definition of the particle flux

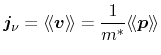$$\ensuremath{\ensuremath{\mathitbf{j}}_\nu}= \ensuremath{\langle \... ...h{\langle \! \langle \ensuremath{\ensuremath{\mathitbf{p}}} \rangle \! \rangle}$$ (3.58)

results in the final isotropic particle flux equation obtained using a power-law approximation for the microscopic relaxation time, parabolic bands and a heated, displaced Maxwellian

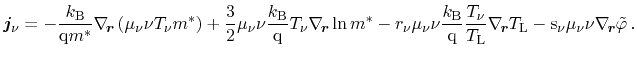$$\ensuremath{\ensuremath{\mathitbf{j}}_\nu}= -\frac{k_\ensuremath{... ...nsuremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}}\ensuremath{\tilde{\varphi}}\,.$$ (3.59)

An alternative formulation of the current equation is obtained by combining the gradients of the carrier concentration $\ensuremath{\nu}$ and the effective mass $m^*$ to the chemical potential which itself is summed up with the electrostatic potential $\ensuremath{\tilde{\varphi}}$ to the electrochemical potential $\ensuremath{\ensuremath{\Phi}_{\ensuremath{\nu}}}$ . Therefore, the effective density of states is introduced [89], which is proportional to $m^*$ and $\ensuremath{T}$

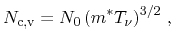$$\ensuremath{N_\mathrm{c,v}}= \ensuremath{N_\mathrm{0}}\left( m^*\ensuremath{T_\ensuremath{\nu}}\right)^{3/2} \,,$$ (3.60)

where $\ensuremath{N_\mathrm{0}}$ reads for a Maxwellian distribution function

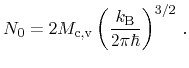$$\ensuremath{N_\mathrm{0}}= 2 \ensuremath{M_\mathrm{c,v}}\left( \frac{k_\ensuremath{\mathrm{B}}}{2 \pi \hbar} \right)^{3/2} \,.$$ (3.61)

The chemical potential $\ensuremath{\ensuremath{\Phi}_{\ensuremath{\nu}}^\mathrm{c}}$ and the electrochemical potential $\ensuremath{\ensuremath{\Phi}_{\ensuremath{\nu}}}$ are introduced as

$$\ensuremath{\ensuremath{\Phi}_{\ensuremath{\nu}}}= \ensuremath{\t... ...\nu}}}{\mathrm{q}} \ln \frac{\ensuremath{\nu}}{\ensuremath{N_\mathrm{c,v}}} \,.$$ (3.62)

Applying Eqs. (3.60) and (3.62) to the particle current (3.59) yields

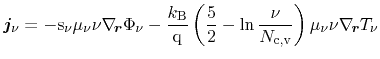$$\ensuremath{\ensuremath{\mathitbf{j}}_\nu}= - \ensuremath{\mathrm... ...nsuremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}}\ensuremath{T_\ensuremath{\nu}}$$ (3.63)

$$\quad - \frac{k_\ensuremath{\mathrm{B}}\ensuremath{T_\ensuremath{... ...ensuremath{\ensuremath{\mathitbf{\nabla_{\!r}}}}}\ensuremath{T_{\mathrm{L}}}\,.$$

M. Wagner: Simulation of Thermoelectric Devices