2.6.1 Electron-Phonon Matrix Elements

An important case is the intra-subband scattering of electrons, $\nu'=\nu$, therefore, $\mu =0$ (Fig. 2.11-b) and $\lambda$ can be any of six different phonon polarizations. One can omit the index $\mu$ and write the phonon frequency as $\omega_{\lambda}(q)$ and the reduced electron-phonon matrix element for a given band as $\widetilde{M}_{k^\prime,\nu^\prime,k,\nu,\lambda}=\widetilde{M}_{\lambda}(q)$, where the weak dependence on $k$ is neglected.

For intra-valley processes, most of the phonons have $q\approx 0$ and are referred to as $\Gamma$-point phonons. Near the $\Gamma$ point a linear dispersion relation for acoustic phonons is assumed,

$$\omega_\mathrm{AP}(q)\ \approx \ \upsilon_\mathrm{AP}\vert q\vert \ ,$$ (2.16)

where $\upsilon_\mathrm{AP}$ is the acoustic phonon velocity. For optical phonons the energy is assumed to be independent of the phonon wave-vector

$$\omega_\mathrm{OP}(q)\ \approx \ \omega_\mathrm{OP} \ .$$ (2.17)

Near the $\Gamma$-point the reduced electron-phonon matrix elements can be approximated by

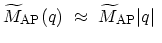$$\widetilde{M}_\mathrm{AP}(q)\ \approx \ \widetilde{M}_\mathrm{AP}\vert q\vert \$$ (2.18)

for acoustic phonons and by

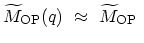$$\widetilde{M}_\mathrm{OP}(q)\ \approx \ \widetilde{M}_\mathrm{OP} \$$ (2.19)

for optical phonons [56]. Phonons inducing inter-valley processes have a wave-vector of $\vert q\vert\approx q_\mathrm{K}$, where $q_\mathrm{K}$ is a wave-number corresponding to the $\mathrm{K}$-point of the Brillouin zone of graphite. For such phonons one can neglect the q-dependence, $\omega_\mathrm{K}(q) \approx \omega_\mathrm{K}$ and $\widetilde{M}_\mathrm{K}(q)\approx \widetilde{M}_\mathrm{K}$ [56].

To calculate the electron-phonon matrix elements one can employ the orthogonal tight-binding [57], the non-orthogonal tight-binding [56], and density functional theory [58] for the band-structure and a force constant model for the lattice dynamics [59,12]. Electron-phonon matrix elements depend on the chirality and the diameter of the CNT [57,56,58]. Figure 2.12 shows the reduced matrix elements for intra-subband intra-valley transitions in semiconducting zigzag and chiral CNTs as a function of the CNT radius [56].

Figure 2.12: The calculated intra-valley intra-subband reduced electron-phonon matrix elements of acoustic (in eV) and optical phonons (in eV/Å) for zigzag (open circles) and chiral (closed) CNTs with the radius range from 3.5 Å to 12 Å. The results for CNTs with residuals 1 and 2 of the division $n-m$ by 3 are shown in the left and right figures, respectively. Open and closed circles denote the results for zigzag (Z) and chiral (C) CNTs, respectively. All results are for the lowest conduction band [56].

M. Pourfath: Numerical Study of Quantum Transport in Carbon Nanotube-Based Transistors