3.8.2 KELDYSH Formulation

For certain applications in classical transport theory it is advantageous to write the BOLTZMANN equation as an integral equation, rather than an integro-differential equation. An analogous situation holds in quantum kinetics. Instead of working with the KADANOFF-BAYM equations (3.62) and (3.63), it may be useful to consider their integral forms. Historically, KELDYSH [202] derived his alternative form almost simultaneously and independently of KADANOFF and BAYM. However, the KELDYSH and KADANOFF-BAYM formalisms are equivalent.

By applying LANGRETH's rules to the DYSON equation (3.44) one obtains

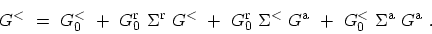$$\begin{array}{l}\displaystyle G^< \ = \ G_0^< \ + \ G_0^\mat... ...}\ + \ G_0^<\ \Sigma^\mathrm{a}\ G^\mathrm{a} \ . \end{array}$$ (3.66)

For convenience a notation where a product of two terms is interpreted as a matrix product in the internal variables (space, time, etc.) has been used. One can proceed by iteration with respect to $G^<$. Iterating once, and regrouping the terms one obtains

$$\begin{array}{ll}\displaystyle G^< \ = \ &\displaystyle \lef... ...rm{r}\ G_0^\mathrm{r}\ \Sigma^\mathrm{r}\ G^< \ . \end{array}$$ (3.67)

The form of (3.67) suggests that infinite order iterations results in [185]

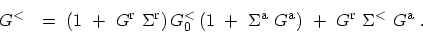$$\begin{array}{l}\displaystyle G^< \ \ = \ \left(1 \ + \ G^\m... ...t) \ + \ G^\mathrm{r}\ \Sigma^< \ G^\mathrm{a}\ . \end{array}$$ (3.68)

Equation (3.68) is equivalent to KELDYSH's results. In the original work, however, it was written for another function, $G_K\equiv G^<+G^>$. This difference is only of minor significance [185].

The first term on the right hand-side of (3.68) accounts for the initial conditions. One can show that this term vanishes for steady-state systems, if the system was in a non-interacting state in the infinite past [185]. Thus, in many applications it is sufficient to only keep the second term.

Similar steps can be followed to obtain the kinetic equation for $G>$. In integral form these equations can be written as

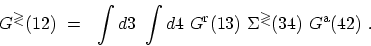$$\begin{array}{ll}\displaystyle G^\mathrm{\gtrless}(12) \ = \... ...igma^\mathrm{\gtrless}(34) \ G^\mathrm{a}(42) \ . \end{array}$$ (3.69)

The relation between the KELDYSH equation and the KADANOFF-BAYM equation is analogous to the relation between an ordinary differential equation plus a boundary condition and the corresponding integral equation.

M. Pourfath: Numerical Study of Quantum Transport in Carbon Nanotube-Based Transistors