3.5.1 The WENTZEL-KRAMERS-BRILLOUIN Approximation

The WENTZEL-KRAMERS-BRILLOUIN^3.6 (WKB) approximation is one of the most frequently encountered assumptions for the quantum-mechanical wave function. It is often used for tunneling simulations and has been implemented in device simulators [128,129,96]. Within the WKB approximation, the transmission coefficient can be written as (for a detailed derivation see Appendix B) [130,131]

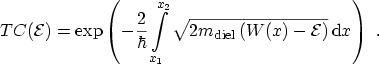$$TC({\mathcal{E}})=\exp\left(-\frac{2}{\hbar}\int_{x_1}^{x_2} \sqr... ...el}}\left( W(x) - {\mathcal{E}}\right) } \,\ensuremath {\mathrm{d}}x\right) \ .$$ (3.55)

In this expression the integration is performed only within the classical turning points $x_1$ and $x_2$, defined by the region where ${\mathcal{E}}\le W(x)$ and the integrand in (3.55) is real. Thus, only the decaying part of the wave function is considered. For a linear energy barrier the numerical calculation of the integral in (3.55) can be avoided. Still, it is necessary to distinguish between regions where direct or FOWLER-NORDHEIM tunneling takes place. For the direct tunneling regime ${\mathcal{E}}< \ensuremath {\mathrm{q}}\Phi_0$ holds (see Fig. 3.9). Therefore, the transmission coefficient

$$TC({\mathcal{E}})=\exp\left(-\frac{2}{\hbar}\int_{0}^{\ensuremath... ...th{E_\mathrm{diel}}x - {\mathcal{E}}\right)} \,\ensuremath {\mathrm{d}}x\right)$$ (3.56)

evaluates to

$$TC({\mathcal{E}}) = \exp\left(-4\frac{\sqrt{2\ensuremath{t_\mathr... .../2} - (\ensuremath {\mathrm{q}}\Phi_0 - {\mathcal{E}})^{3/2}\right) \right) \ ,$$ (3.57)

with $\ensuremath{E_\mathrm{diel}}$ being the electric field defined as $\ensuremath{V_\mathrm{diel}}/\ensuremath{t_\mathrm{diel}}$ and $\ensuremath{m_\mathrm{diel}}$ the electron mass in the dielectric. The symbols $\Phi$ and $\Phi_0$ denote the upper and lower barrier heights, as shown in Fig. 3.9. The value of $\Phi_0$ is calculated assuming a linear potential in the barrier

$$\Phi_0 = \Phi - \ensuremath{E_\mathrm{diel}}\ensuremath{t_\mathrm{diel}}\ .$$ (3.58)

For the FOWLER-NORDHEIM tunneling regime it holds ${\mathcal{E}}> \ensuremath {\mathrm{q}}\Phi_0$ and therefore with $x_1$ defined by $\ensuremath {\mathrm{q}}\Phi - \ensuremath {\mathrm{q}}\ensuremath{E_\mathrm{diel}}x_1 = {\mathcal{E}}$ the transmission coefficient

$$TC({\mathcal{E}})=\exp\left(-\frac{2}{\hbar}\int_{0}^{x_1} \sqrt{... ..._\mathrm{diel}}x - {\mathcal{E}}\right)} \,\ensuremath {\mathrm{d}}x\right) \ ,$$ (3.59)

evaluates to

$$TC({\mathcal{E}}) = \exp\left(-4\frac{\sqrt{2\ensuremath{m_\mathr... ..._\mathrm{diel}}} (\ensuremath {\mathrm{q}}\Phi-{\mathcal{E}})^{3/2} \right) \ .$$ (3.60)

The WKB tunneling coefficient is frequently multiplied by an oscillating prefactor to reproduce FOWLER-NORDHEIM-induced oscillations [132,133,134,135,136]. However, since no wave function interference is taken into account, the general validity of this method is questionable.

A. Gehring: Simulation of Tunneling in Semiconductor Devices