3.7 Compact Tunneling Models

The above presented models for the calculation of tunneling currents require a considerable computational effort. However, for practical device simulation, it is desirable to use compact models which do not require large computational resources. That may be necessary for a quick estimation of the dielectric thickness from IV data or to predict the impact of gate leakage on the performance of CMOS circuits [178,179,180,181,182,183]. The most frequently used model to describe tunneling is the FOWLER-NORDHEIM formula [184]

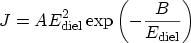$$J= A \ensuremath{E_\mathrm{diel}}^2 \exp \left( -\frac {B} {\ensuremath{E_\mathrm{diel}}} \right)$$ (3.117)

which was originally used to describe tunneling between metals under intense electric fields. The parameters $A$ and $B$ have been refined by LENZLINGER and SNOW [185]:

$$J= \frac {\ensuremath {\mathrm{q}}^3\ensuremath{m_\mathrm{eff}}} ... ...})^3}} {3\hbar \ensuremath {\mathrm{q}}\ensuremath{E_\mathrm{diel}}} \right)\ .$$ (3.118)

This expression can be derived from the TSU-ESAKI formula (3.13) by the assumption of zero temperature, a triangular energy barrier, and equal materials on both sides of the dielectric (the derivation is shown in Appendix A). Thus, it is not valid for direct tunneling where the barrier is of trapezoidal shape. Furthermore, $\ensuremath {\mathrm{q}}\ensuremath{\Phi_\mathrm{B}}$ denotes the difference between the FERMI energy in the electrode and the conduction band edge in the dielectric, and not the conduction band offset, as it is often found in the literature.

SCHUEGRAF and HU derived correction terms for this expression to make it applicable to the regime of direct tunneling [186]

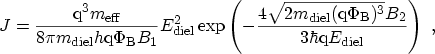$$J= \frac {\ensuremath {\mathrm{q}}^3\ensuremath{m_\mathrm{eff}}} ... ... B_2} {3\hbar \ensuremath {\mathrm{q}}\ensuremath{E_\mathrm{diel}}} \right) \ ,$$ (3.119)

with the correction terms $B_1$ and $B_2$ given as (the derivation can also be found in Appendix A)

$$\displaystyle B_1 = \left( 1 - \left(1 - \frac{\ensuremath {\math... ...\ensuremath {\mathrm{q}}\ensuremath{\Phi_\mathrm{B}}}\right)^{1/2}\right)^2 \ ,$$ (3.120)

and

$$\displaystyle B_2 = \left( 1 - \left(1 - \frac{\ensuremath {\math... ...}}{\ensuremath {\mathrm{q}}\ensuremath{\Phi_\mathrm{B}}}\right)^{3/2}\right)\ .$$ (3.121)

For a triangular barrier the correction factors become $B_1=B_2=1$ and the expression simplifies to (3.118). Note that using these equations, the minimum tunneling current occurs for $\ensuremath{E_\mathrm{diel}}=0$V/m which, for a work function difference $\neq 0$, does not occur at the minimum applied bias.

A. Gehring: Simulation of Tunneling in Semiconductor Devices