3.3.2.2 Average Nuclear Stopping Power

Although it is not relevant for the Monte-Carlo simulation of ion implantation it is quite interesting to make some statements of the average nuclear stopping power.

Knowing the interatomic potential it is possible to derive the average nuclear stopping power $S_n(E_0)$ which defines the average energy that is transfered by each nuclear collisions if the particle energy is $E_0$. $S_n(E_0)$ can be calculated by integrating the energy transfer $T(E_0,\theta(p,E_C))$ of a nuclear collision event over all possible impact parameters $p$.

$$\begin{split}S_n(E_0) &= \int\limits_{0}^{\infty} T(E_0,\thet... ...t(\frac{\theta(p,E_C)}{2}\right) \cdot p \;\cdot dp \end{split}$$ (3.93)

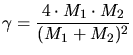$$\gamma = \frac{4\cdot M_1\cdot M_2}{(M_1+M_2)^2}$$ (3.94)

Although there is no analytical solution for the average nuclear stopping power $S_n(E_0)$, a numerical solution can be derived using equations (3.90), (3.89) and (3.88) for the scattering angle $\theta(p,E_C)$ [92]. Therefore the so called dimensionless reduced energy $\epsilon$ and the dimensionless reduced nuclear stopping have to be introduced.

The reduced energy considers the dependence of the nuclear stopping power on the masses ($M_1$ and $M_2$) and the charges ($Z_1$ and $Z_2$) of the atoms involved in the scattering process. The relation between the real ion energy $E_0$ in units of keV and the reduced energy is given by

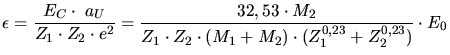$$\epsilon = \frac{E_C\cdot \;a_U}{Z_1\cdot Z_2\cdot e^2}=\frac{32,53\cdot M_2}{Z_1\cdot Z_2\cdot (M_1+M_2)\cdot (Z_1^{0,23}+Z_2^{0,23})}\cdot E_0$$ (3.95)

This relation is also plotted in in Fig. 3.6 for various atom pairs relevant for semiconductor ion implantation to give an impression of the order of the reduced energy.

Figure 3.6: Reduced energy as function of the ion energy.

The reduced nuclear stopping is defined by

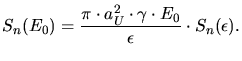$$S_n(E_0) = \frac{\pi \cdot a_U^2\cdot \gamma \cdot E_0}{\epsilon}\cdot S_n(\epsilon).$$ (3.96)

It allows to find an analytical approximation to the numerical solution of the nuclear stopping power. The analytical approximation for $S_n(\epsilon)$ is given by (3.97) and plotted in Fig. 3.7 [92].

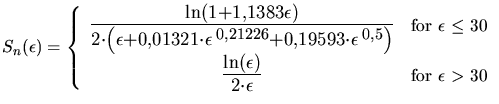$$S_n(\epsilon) = \LARGE\left\{ \begin{array}{cl} \frac{\ln(1+1,138... ...cdot \epsilon} & \text{\normalsize for $\epsilon$\ $>$\ 30} \end{array} \right.$$ (3.97)

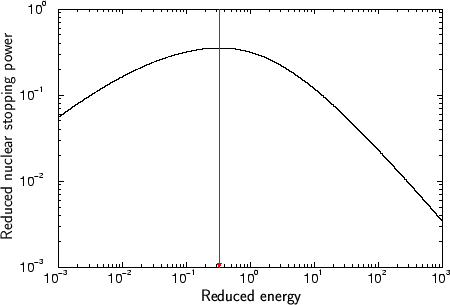
Figure 3.7: Reduced nuclear stopping power as function of the reduced energy.

Applying this approximation to (3.96) gives the nuclear stopping power as expressed in (3.98) in units of eV/(atom/cm$^{2}$), by additionally inserting (3.94), (3.95) and (3.92).

$$\begin{split}S_n(E_0) &= \frac{\pi \cdot a_U^2\cdot \gamma\cd... ...2)\cdot (Z_1^{0,23}+Z_2^{0,23})}\cdot S_n(\epsilon) \end{split}$$ (3.98)

$S_n(\epsilon)$ (3.97) has its maximum at a reduced energy of $\epsilon=$0,327 where also the average nuclear stopping power has its maximum according to (3.98). The relating real particle energy where the average stopping has is maximum can be calculated by (3.95). Tab. 3.2 summarizes the approximate stopping power maxima for various atom pairs.

Table 3.2: Maxima of the average nuclear stopping power for various atom pairs.

Incident particle	Target particle	Nuclear stopping power maximum (keV)
Boron	Silicon	3
Phosphorus	Silicon	16
Arsenic	Silicon	68
Antimony	Silicon	160
Boron	Oxygen	2
Phosphorus	Oxygen	12
Arsenic	Oxygen	58
Antimony	Oxygen	143