3.3.4.1 The Ohmic Contact: Electrical Treatment

The potential at the conventional Ohmic contact interface is calculated in a local standard model. The assumed condition for the potential reads:

$$\varphi_s = \varphi_m +\psi_{bi}$$ (3.99)

with $\varphi_s$ the contact potential, $\varphi_m$ the metal quasi Fermi-level equivalent to the applied contact voltage, and $\psi_{bi}$ the built-in potential. (3.102) represents a Dirichlet condition. The built-in potential equals the potential caused by the Fermi level adjustment at any interface. This potential reads, as given in [90]:

$$\psi_{bi} = \frac{{\it k}_{\mathrm{B}}\cdot {\it T}_\mathrm{L}}{q}\cdot \ln\bigg(\frac{1}{2\cdot C_1}\cdot \Big( C+ \sqrt{C^2+4\cdot C_1\cdot C_2}\Big)\bigg)$$ (3.100)

$$ = - \frac{{\it k}_{\mathrm{B}}\cdot {\it T}_\mathrm{L}}{q}\cdot \l... ...gg(\frac{1}{2\cdot C_2}\cdot \Big(-C+ \sqrt{C^2+4\cdot C_1\cdot C_2}\Big)\bigg)$$ (3.101)

$C$ is the overall net concentration of the applied doping at the boundary. The auxiliary coefficients in (3.104) are defined as:

$$C_1 = N_C \cdot \exp  \bigg(\frac{-E_C}{{\it k}_{\mathrm{B}}\cdot {\it T}_\mathrm{L}} \bigg)$$ (3.102)

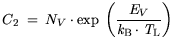$$C_2 = N_V \cdot \exp  \bigg(\frac{-E_V}{{\it k}_{\mathrm{B}}\cdot {\it T}_\mathrm{L}} \bigg)$$ (3.103)

The carrier concentrations in the semiconductor at the boundary are pinned to the equilibrium concentrations at the contact, which read:

$$n_s = N_C \cdot \exp \bigg( \frac{-E_C+q \cdot \psi_{bi}}{{\it k}_{\mathrm{B}}\cdot {\it T}_\mathrm{L}}\bigg)$$ (3.104)

and:

$$p_s = N_V \cdot \exp \bigg(\frac{ E_V- q \cdot \psi_{bi}}{{\it k}_{\mathrm{B}}\cdot {\it T}_\mathrm{L}}\bigg)$$ (3.105)

This assumes a high doping of the semiconductor of the order of ${\it N}_{\mathrm{D}}\geq$ 10$^{18}$ cm$^{-3}$. Any specific interface effects, such as dipole charges, surface charges, or a resulting semiconductor-metal alloy, are neglected. The carrier temperatures ${\it T}_\mathrm{\nu}$ at the Ohmic contact are modeled as:

$${\it T}_\mathrm{L} = T_{\nu}$$ (3.106)

with ${\it T}_\mathrm{L}$ the lattice temperature at the contact, i.e., the carriers enter the semiconductor in thermal equilibrium. A finite electrical line resistance of the contact metal $R_C$ can be included using:

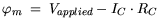$$\varphi_{m} = V_{applied}-I_{C}\cdot R_{C}$$ (3.107)

with $V_{applied}$ the applied terminal voltage, and $I_{C}$ the current through the contact.